Biconic Approximation of a Toric Surface

Wei-Jun Chen

R&D Biometry, Ophthalmology, Carl Zeiss Meditec AG, G

oschwitzer Straße 51–52, Jena, Germany

Keywords:

Biconic Model, Toric Lens, Sphere, Cylinder, Ellipsoid, Elliptic Cylinder, Astigmatism, Approximation.

Abstract:

In this work, a toric surface is explicitly approximated by a biconic model by decomposing the toric surface

into a biconic–identical component and other elliptical cylinder–related residual components. These residual

components determine the zone– and the azimuthal orientation–dependent approximation accuracy. In addi-

tion to the analytical underpinning, a direct fit of the biconic model to a data set sampled from a toric surface

is performed and consistent results are obtained.

1 INTRODUCTION

In geometrical optics as well as in ophthalmology

(cornea), an optical surface with the dominant opti-

cal aberration of astigmatism is usually called a toric

surface, which has two different refractive powers (re-

fractive curvatures) in two perpendicular meridians,

resulting in individual focal lengths.

There are three different but often intermingled

descriptions of a toric surface: 1, an optical surface

as a “cap” of a torus (Wiki-article, 2023); 2, an op-

tical surface as a composite “spherical + cylindrical”

surface (Barcala et al., 1995); 3, an optical surface de-

scribed by a biconic model with two different radii in

two perpendicular axes (e.g., the x– and y–axes).

In general, the first description of the “cap” of a

torus is often used to theoretically explain the geo-

metric properties of a toric surface, with the precise

mathematical definition being more visually under-

stood (Bartkowska, 1998) (Krasauskas, 2001), while

the second description is more of an industry– and

market–oriented description that provides an intuitive

understanding of the optical behavior of a toric lens.

The third description is a more engineering–oriented

description that is commonly used for optical design

and aberration compensation (Guo and Sun, 2017),

lens manufacturing (Chen et al., 2011), clinical tri-

als (P

erez-Escudero et al., 2010) (Janunts et al., 2015)

(Moore et al., 2019) (Giraudet et al., 2022) (Lan-

genbucher et al., 2023), scientific research in vari-

ous fields (Einighammer, 2008) (Gatinel et al., 2011)

(Pi

nero et al., 2012) (Navarro et al., 2019) (Con-

sejo et al., 2021), etc. In addition, optical surfaces

with higher complexity, such as aspheres and higher–

order freeform surfaces, are often designed based on

a toric or biconic model plus additional conical con-

stants and individual Zernike components (Meister,

1998) (Roffman and Menezes, 1998) (Rosales et al.,

2009) (Scholz et al., 2009) (Gu et al., 2019) (Br

omel,

2018) (Volatier et al., 2020).

Although the three descriptions above differ for-

mally, and have more or less their own flavor, they

attempt to provide consistent information about the

same optical aberration of astigmatism based on a

well–accepted assertion: For a true toric surface, a

biconic model with well–determined parameters is a

good approximation (Navarro, 2009). Nevertheless, it

is not so easy to find in the literature a direct answer

to the following question, namely how good such an

approximation is, for different specific applications.

An example of this can be found in Fig. 1, where

the comparable astigmatism of a toric and a biconic

model is illustrated by their refractive power maps

in the 12–mm zone

. However, the toric model also

has somewhat “asphericity” inside, while this is not

true for the biconic model, although these two models

have identical optical and geometric parameters.

With explicitly defined parameters of radius (r

)

and astigmatism (δ) for spherical and cylindrical sur-

faces, mathematical definitions of the toric surface

and the biconic model are rewritten in this paper.

Moreover, they are compared and decomposed into

their identical part and their distinct components,

where these distinct components are analogous to sev-

eral sums of polynomials of the astigmatism defined

elliptic cylinder, which explain well the intrinsic as-

The 12–mm and 6–mm zones are two typically relevant

zones in refractive laser surgery and cataract surgery.

Chen, W.

Biconic Approximation of a Toric Surface.

DOI: 10.5220/0012336800003651

Paper published under CC license (CC BY-NC-ND 4.0)

In Proceedings of the 12th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2024), pages 45-50

ISBN: 978-989-758-686-6; ISSN: 2184-4364

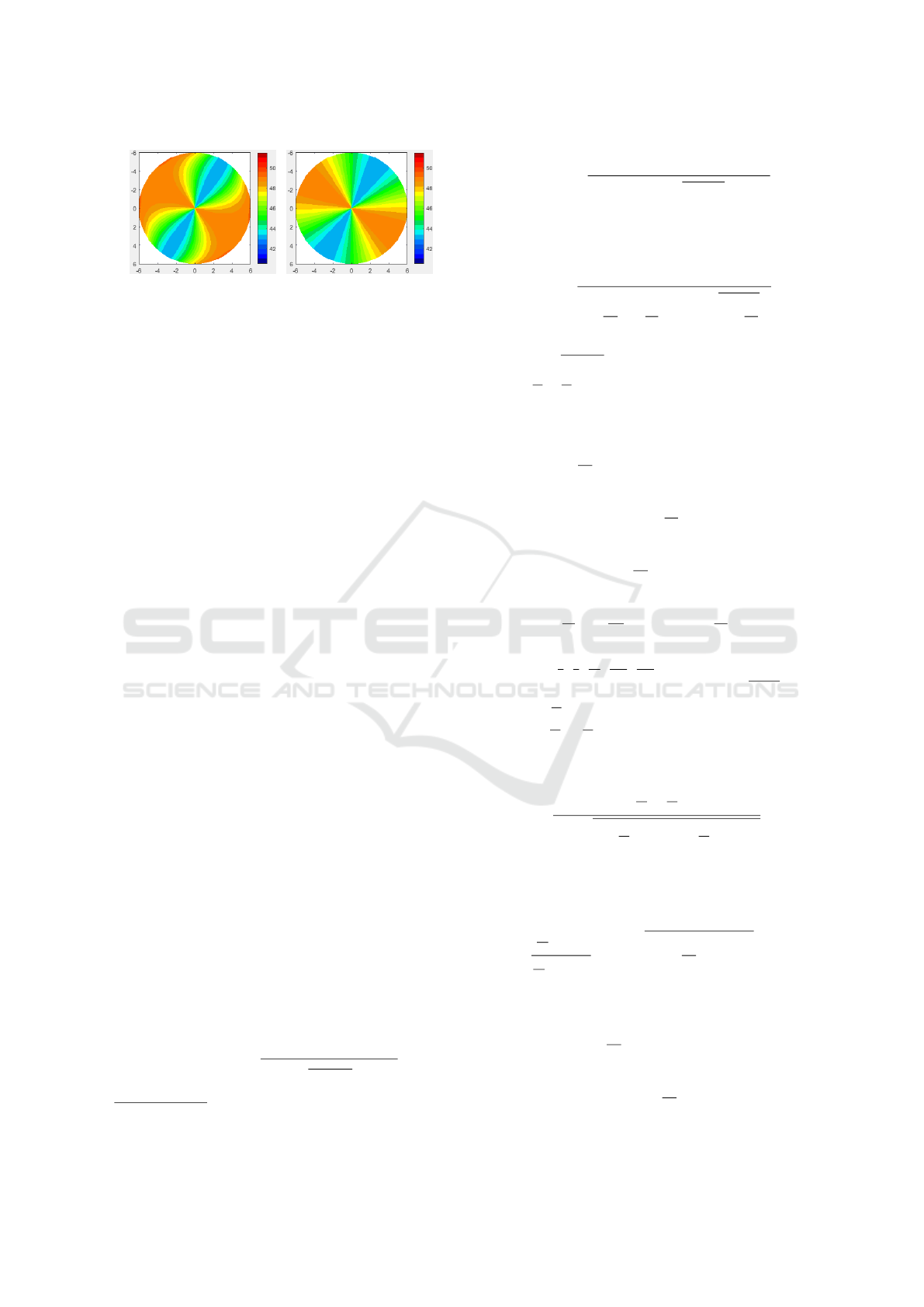
(a) (b)

Figure 1: Refractive power maps of: (a) Toric model; (b)

Biconic model. Model parameters: r

= 6.88 mm, r

7.85 mm, cylinder axis = 31

◦

, refractive index = 1.3375,

map zone = 12 mm, map color unit = D (diopter).

phericity of a standard toric surface, and analytically

determine the quality of approximation of a biconic

model to a toric surface in a simple way.

The rest of this paper is organized as follows:

Sec. 2 rewrites the toric surface and the biconic model

with explicit optical parameters; Sec. 3 compares

these two models and decomposes them; the quality

of approximation of a biconic model to a toric sur-

face is first evaluated analytically in Sec. 4, and then

verified in Sec.4.1 by directly fitting a biconic model

to a discrete sample set taken from a predefined toric

surface; Sec. 5 discusses the evaluation results; finally

Sec. 6 concludes this paper with an expectation for fu-

ture work.

2 TORIC AND BICONIC MODELS

The standard toric surface as “cap” of a torus could be

defined by parametric equations











x = (c + a cosv)cosu

y = (c + a cosv)sinu

z = a sin v

(1)

describing a surface of revolution generated by the ro-

tation of a circle in 3D about the z–axis, where a de-

notes the radius of the circle, c the decentering of the

circle center from the z–axis, v the angular parameter

of the points on the circle, and u the angle of rotation.

If we move the coordinate origin to an optical ver-

tex on the surface from (x, y, z) →(x, y−(c+a).z) and

swap the axes y and z as (x, y, z ) → (x, −z, y), we get











x = (c + a cosv)cos u

z = (c + a) −(c + acosv) sin u

y = a sin v

(2)

which corresponds to

z = (c + a) −



c +

−y



−x

(3)

https://mathworld.wolfram.com/Torus.html

and in turn can be rewritten as

z = r

−



−r

) +

−y



−x

(4)

where two radii are defined as r

= c + a and r

= a.

Extracting r

and r

from two squared root compo-

nents, we obtain

z = r

1 −

+ 2



1 −



(5)

where h =

+ y

is the polar radius in the xy plane,

and δ =

−

is the astigmatism of the toric surface.

If we continue the Taylor expansion for two

squared root components in Eq. 5, we obtain:

z =

−δy

−2δ



∞

∑

k=1

k+1







∞

∑

m=0

m+1

+ ∆

−

2δ



∞

∑

k=1

k+1







(6)

where p

128

256

, ... for m = 1, 2, 3, 4, 5, ...

are polynomial coefficients of f (x) = 1 −

√

1 −x, and

∆

= δ(δ −

) is an astigmatism dependent factor de-

rived from

+ ∆

On the other hand, the standard biconic model is

defined as

z =

1 +

1 −

(1 + k

) −

(1 + k

)

(7)

where k

and k

denote two conic constants and are

all zero when only astigmatism is considered. With

the above h, δ and ∆

, Eq. 7 is equivalent to

z =

−δy

+ ∆

1 −



+ ∆



(8)

which has also its polynomial version:

z =

−δy

∞

∑

m=0

m+1

+ ∆

. (9)

PHOTOPTICS 2024 - 12th International Conference on Photonics, Optics and Laser Technology

3 MODEL COMPARISON

Comparing Eq. 6 and Eq. 9, we see that the standard

toric surface as a “cap” of a torus contains a biconic

part. The Eq. 6 is then rewritten into

z =

−E

∞

∑

m=0

m+1

∆

−

(10)

with

−δy

(11)

∆

+ ∆

(12)

C = δy

(13)

E = 2C



∞

∑

k=1

k+1







(14)

where H

and H

∆

contribute to the multiplier and the

multiplicand in the biconic model (Eq. 9), and C and

E denote a cylinder and an elliptical–cylinder, respec-

tively, inside the toric surface.

4 APPROXIMATION QUALITY

From the above Eqs.10–14, the approximation qual-

ity of a biconic model to a toric surface is determined

by the cylinder and elliptical–cylinder components in-

side a toric surface. If we subtract the biconic part

from a toric surface, we get the remainder as

rem

= z

toric

−z

biconic



−E





∞

∑

m=1

∑

n=1

m+1

m−n

∆



−





−



∞

∑

m=0

m+1

∆



(15)

where b

denotes individual binomial coefficients.

The approximation error, i.e., the difference be-

tween a biconic model and its approximated toric sur-

face, can be calculated by Eq. 15. To facilitate the

analysis of zones and azimuthal orientation, the term

is replaced by h

sin

(ρ+ϕ) in all equations above,

where (h, ρ) denotes the polar coordinates of 2D po-

sitions in xy plane and ϕ the axis orientation of the

astigmatism. In the following analysis, the example

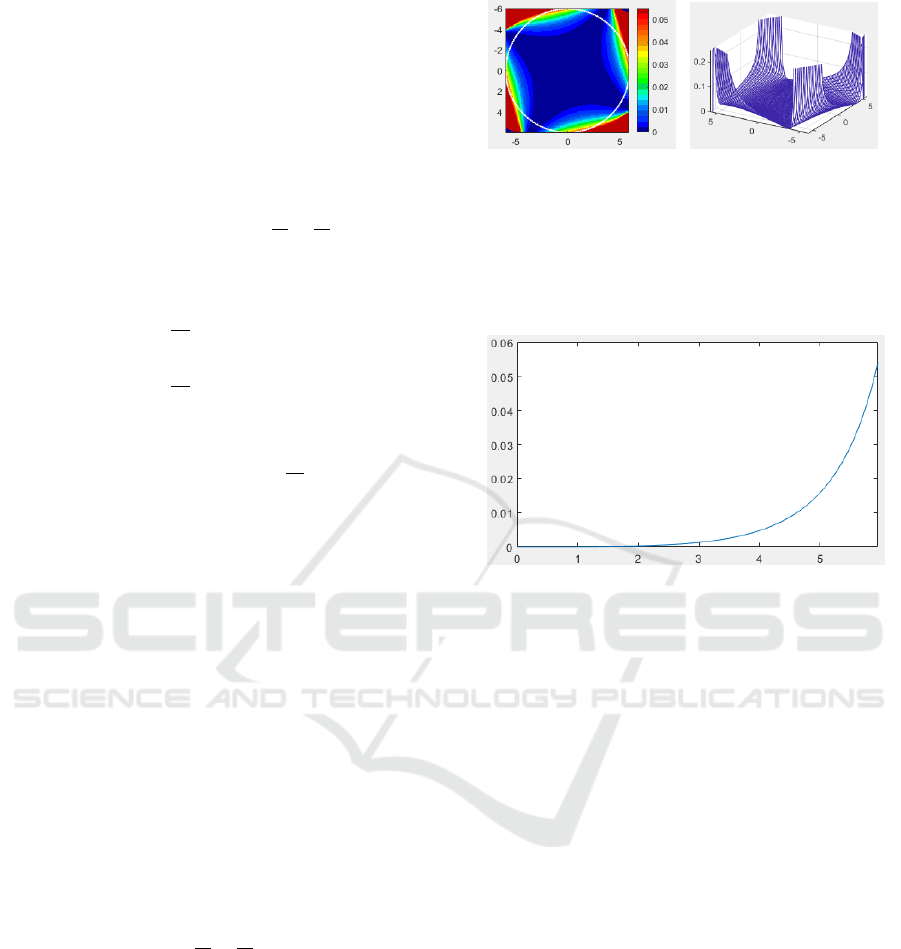
(a) (b)

Figure 2: Approximation error as the difference between

a true toric surface and its biconic approximation: (a)

Differential map z

rem

, where the color map illustrates the

value range from 0 to 0.05mm; (b) 3D mesh visualization

of the approximation error, where the value range in z axis

is from 0 to 0.25mm.

Figure 3: Zone dependence of the approximation error:

the curve of z

max

rem

(h) = max



rem

(h, ρ)|h ∈ [0 6mm], ρ ∈

◦

360

◦

)



shown in Fig. 1 is evaluated using Eq. 15, and the ap-

proximation error is first illustrated in Fig. 2 for z

rem

over the whole 12 mm ×12 mm region.

The approximation error of a biconic model to a

true toric surface is zone dependent and also depends

on the azimuthal orientation. Using the model pa-

rameters given in Fig. 1, the zone dependence is il-

lustrated in Fig. 3. From this, it can be seen that

the maximum approximation erorr for the example

in Fig. 1 is ≈ 0.0012371 mm for a 6–mm zone (i.e.,

h ≤ 3 mm). The dependence on the azimuthal orienta-

tion is shown in Fig. 4, where 12 curves of z

rem

(h, ρ)

for individual h values are shown, the horizontal axis

being the azimuthal direction from 0

◦

to 360

◦

. It is

shown that the approximation error of the evaluated

example is lowest along the astigmatism axis ϕ and

its perpendicular axis (i.e., 31

◦

and 121

◦

for the ex-

ample in Fig. 1), while the maximum error is along

the azimuthal orientations of ϕ ±45

◦

The quality of approximation of a biconic model

to a true toric surface is also illustrated by the

differential refractive power map in Fig. 5, from

which we obtain the information that the maximum

approximation errors in the 6–mm zone and the 12–

mm zone are ≈ 0.36D and ≈ 3.22D, respectively.

Biconic Approximation of a Toric Surface

(a)

(b)

Figure 4: Azimuthal orientation dependence of the ap-

proximation error: the curve of z

rem

, ρ) where h

k ×0.47 mm, and ρ ∈ [0

◦

360

◦

): (a); 6 curves for (h

|k =

0, 1, 2, ...5); (b) 6 curves for (h

|k = 6, 7, 8...11).

Figure 5: Evaluation of the approximation error by differen-

tiation of two refractive power maps, with two circles drawn

in the map to indicate the 6–mm zone and the 12–mm zone.

4.1 Biconic Model Fitting

In addition to the analytical evaluation of the qual-

ity of approximation of a biconical model to a given

true toric surface described above, this section de-

scribes an experimental evaluation by fitting a bi-

conic model to a discrete sample set, where the sam-

ple set X = (x

, y

, z

|i = 1, 2, ..., N) contains a total of

16384 points sampled within an 11–mm zone through

a regular 128 ×128 grid with a spatial resolution of

0.09375 mm on the xy plane, and the z–values were

calculated using the standard toric model (Eq. 4) with

model parameters of the example in Fig. 1. Sampling

in the 11–mm zone instead of the 12–mm zone serves

to avoid the surface edge effect.

A non–linear LeastSquares fitting was performed

Table 1: Biconic model fitting results.

Parameters Truth A–ﬁt AC–ﬁt

(mm) 6.88 6.8660 6.8669

(mm) 7.85 7.8260 7.8755

sph

(mm) 7.365 7.346 7.371

Sph (D) 45.8248 45.9434 45.7875

Cyl (D) 6.0616 6.0298 6.2944

ϕ (

◦

) 31

◦

31.0001 30.9997

0 0 -0.0001

0 0 0.0556

RMS (mm) 0 0.00359 0.00321

Max (mm) 0 0.016 0.015

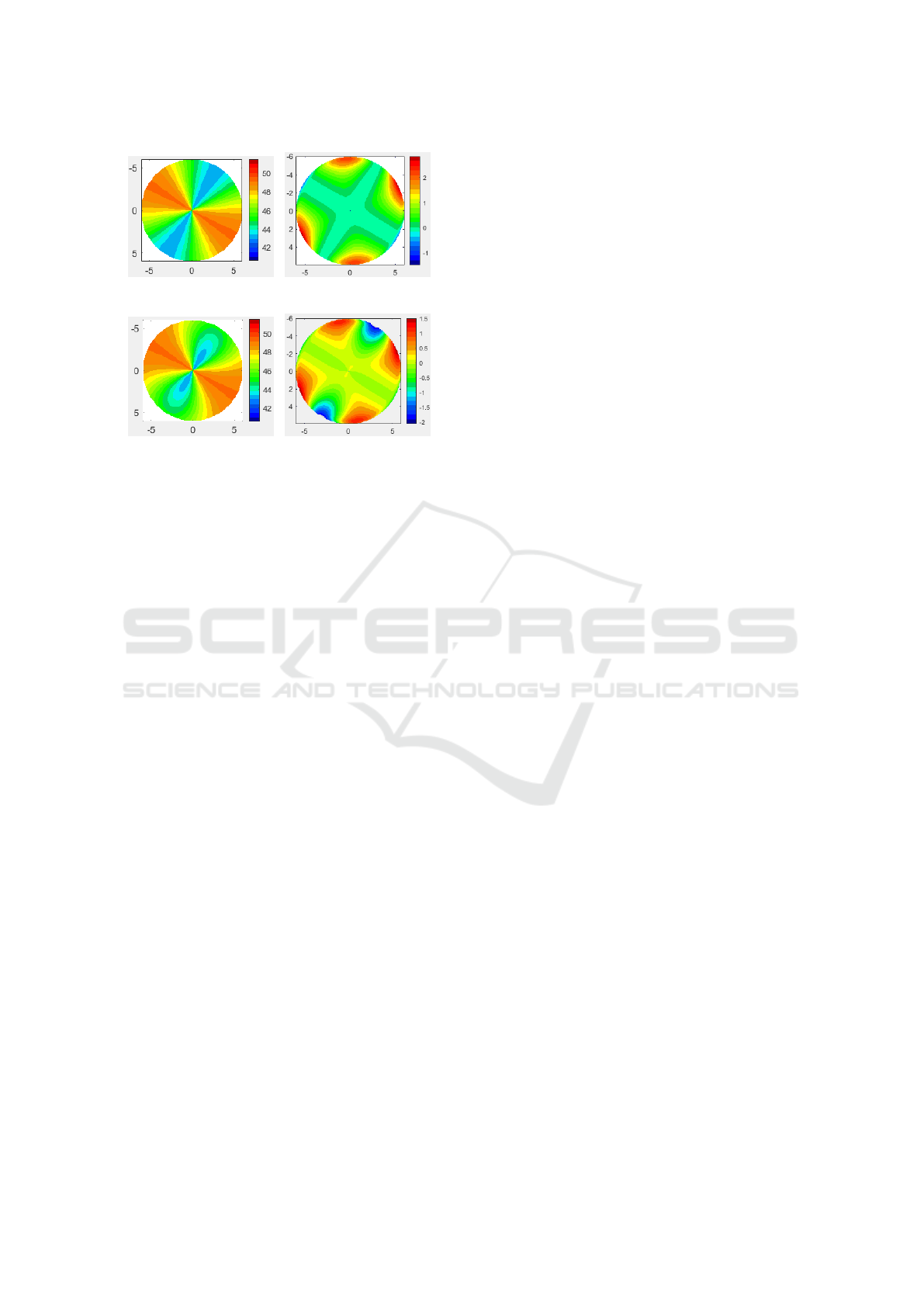
(a) (b)

Figure 6: Fitting error maps: (a) The pure astigmatism fit;

(b) The astigmatism & conic fit.

based on the standard MATLAB optimization func-

tion lsqcurvefit and the standard biconic model

(Eq. 7). Two model fits were performed, one with

the model parameter for (r

, δ, ϕ) as a pure astigma-

tism fit (abbreviation: A–fit), and another with all the

biconical parameters (r

, δ, ϕ, k

, k

), namely an astig-

matism & conic fit (AC–fit).

The results of these two model fits are shown in

Table 1. From this, we can see that the estimation er-

rors of these two fits for the two perpendicular radii

are ≈ −0.014 mm for r

and ≈ ±0.025 mm for r

while the error for the astigmatism axis is quite small

(≈ 0.0002

◦

). Moreover, the AC–fit is slightly bet-

ter than the A–fit, in terms of the RMS criterion, but

all RMS are > 3 µm for the 11–mm zone. Mean-

while, the maximum error in the 11–mm zone is about

0.015 mm, which is consistent with the analytical

evaluation (Fig. 3).

The corresponding 2D error maps for these two

fits are shown in Fig. 6. In contrast to the analytical

evaluation (Fig. 2), these two model fits produce both

positive and negative errors because the least–squares

optimization is a compromise. For a better under-

standing of the fitting results, refractive power maps

of these two model fits are also shown in Fig. 7. From

these two maps, we know that the A-fit has the same

map structure as the theoretical result (Fig. 5), but

with a mean power shift of ≈ +0.12D, which is con-

sistent with the difference of the equivalence sphere

PHOTOPTICS 2024 - 12th International Conference on Photonics, Optics and Laser Technology

(a) (b)

Figure 7: Model fitting results: (a), The refractive power

of the pure astigmatism fit; (b), The differential map as the

fitting residual of (a); (c), The refractive power of the astig-

matism & conic fit; (d), The differential map as the fitting

residual of (c).

(Sph in Table 1). On the other hand, the AC–fit pro-

duces a different power map structure due to the as-

phericity of two conical constants, which is different

from the asphericity resulting from the sums of the

polynomials of an elliptical cylinder on the toric sur-

face (Fig. 1(a)).

5 DISCUSSIONS

Based on the above theoretical and experimental un-

derpinning, approximating a biconic model to a toric

surface within a bounded zone, such as the 6–mm

zone for our example surface is good enough. If we go

beyond such a bounded zone, the approximation error

increases significantly, as shown in Fig. 3 and Fig. 4.

On the other hand, the approximation error is theo-

retically zero along the astigmatism axis and its per-

pendicular direction, while it periodically increases to

a maximum value at ≈ ±45

◦

from the astigmatism

axis. The approximation error is not random noise,

but structural deviation; the random noise is generally

expected to be suppressed by optimising the model

parameters, while the structural deviation is consid-

ered to be compensated by model selection and the

addition/removal of orthogonal components such as

Zernike components.

The interior of a toric surface contains an inher-

ent aspherical component, but it does not seem to

be readily described by conical constants in the bi-

conic model, as shown in Fig. 7(c) and Fig. 7(d).

Moreover, adjusting the conic constant in the biconic

model helps to reduce the RMS very slightly but non–

negligibly increases the approximation error of core

surface descriptors, such as the spherical radius and

the astigmatism, i.e. the K (keratometer) and the Cyl

(cylinder) values in the corneal clinic.

It should be noted that this paper investigates the

quality of approximation between two known mod-

els with available model parameters, which is only a

part of the full solution in many practical applications,

e.g., lens quality inspection, refractive modeling of

human corneal surfaces, etc. The focus of this work

is not to model the measured data by determining op-

timal parameters for a particular model, but rather

to provide a solid basis for the selection of torus or

biconical models with similar model parameters for

different geometries and refractive requirements.

6 CONCLUSIONS

It turns out that the quality of approximation of the

biconical model to a toric surface is good enough

within a bounded zone, while outside such a zone the

approximation error increases considerably. By rep-

resenting the standard toric model as a standard bi-

conic model plus remainder components, the approx-

imation error can be calculated analytically using the

torus parameters of the base sphere radius, astigma-

tism, and astigmatism axis. Meanwhile, these remain-

ing components are connected to an elliptical cylin-

der defined by the toric astigmatism as well as the

radius of the base sphere. This creates an intrinsic

aspheric structure within the toric surface that does

not directly correspond to the ellipsoid–like biconical

structure defined by two conical constants.

This work provides a solid foundation for further

research on the following three topics: First, from

the analysis of the remainder composition (Eq. 15),

the approximation acceptable zone should be explic-

itly determined by the torus parameters of astigma-

tism and base sphere; second, the choice of the model

for given measured data should take into account

different geometric structures under the same model

parameters, such as different aspherical structures in

the toric equation and the biconical equation; third,

it has been shown (Eqs. 6 and 9), but not further in-

vestigated, that both the toric model and the biconic

model are fully compatible with Zernike polynomi-

als. By combining individual Zernike components in

different ways, determining model parameters from

Zernike coefficients should be suitable for both of the

above aspheric structures.

Biconic Approximation of a Toric Surface

ACKNOWLEDGEMENTS

The author would like to thank his colleagues:

Christopher Weth, Tobias Buehen, Ziyao Tang, and

Carol Zhang, for their valuable technical discussions.

REFERENCES

Barcala, J., Vazquez, M. C., and Garcia, A. (1995). Optic

systems with spherical, cylindrical, and toric surfaces.

Applied Optics, 34(22):4900–4906.

Bartkowska, J. (1998). Toroidal surfaces in ophthalmic op-

tics. In Proceedings SPIE: Ophthalmic Measurements

and Optometry, volume 3579, pages 76–93.

omel, A. (2018). Dissertation: Development and

evaluation of freeform surface descriptions.

Friedrich–Schiller-Universit

at Jena, Jena.

Chen, C.-C., Cheng, Y.-C., Hsu, W.-Y., Chou, H.-Y., Wang,

P. J., and Tsai, D. P. (2011). Slow tool servo diamond

turning of optical freeform surface for astigmatic con-

tact lens. In Optical Manufacturing and Testing IX,

volume 8126. Proc. of SPIE.

Consejo, A., Fathy, A., Lopes, B. T., Renato Ambr

osio, J.,

and Abass, A. (2021). Effect of corneal tilt on the

determination of asphericity. Sensors, 21(22):1–15.

Einighammer, J. (2008). Dissertation: The Individual Eye.

Eberhard-Karls-Universit

at T

ubingen, T

ubingen.

Gatinel, D., Malet, J., Hoang-Xuan, T., and Azar, D. T.

(2011). Corneal elevation topography: Best ﬁt sphere,

elevation distance, asphericity, toricity and clinical

implications. Cornea, 30(5):508–515.

Giraudet, C., Diaz, J., Tallec1, P. L., and Allain1, J.-

M. (2022). Multiscale mechanical model based on

patient-speciﬁc geometry: application to early kera-

toconus development. Journal of the Mechanical Be-

havior of Biomedical Materials, 129:1–13.

Gu, Y., McKenney, C. D., and Niculas, C. D. (2019).

Method for manufacturing toric contact lenese. US

Patent Application: US 2019/0193350 A1.

Guo, Y. and Sun, L. (2017). Biconic white multipass cell

design based on a skew ray–tracing model. Applied

Optics, 56(27):7586–7595.

Janunts, E., Kannengießer, M., and Langenbucher, A.

(2015). Parametric ﬁtting of corneal height data to

a biconic surface. Zeitschrift f

ur Medizinische Physik,

25(1):25–35.

Krasauskas, R. (2001). Shape of toric surfaces. In Proceed-

ings Spring Conference on Computer Graphics, pages

55–62.

Langenbucher, A., Szentm

ary, N., Cayless, A., Muen-

ninghoff, L., Wylegala, A., Wendelstein, J., and Hoff-

mann, P. (2023). Bootstrapping of corneal optical co-

herence tomography data to investigate conic ﬁt ro-

bustness. Journal of Clinical Medicine, 12:1–16.

Meister, D. (1998). Principles of atoric lens design. Lens

Talk, 27(03):1–4.

Moore, J., Shu, X., Lopes, B. T., Wu, R., and Abass, A.

(2019). Limbus misrepresentation in parametric eye

models. PLoS ONE, 15(9):1–22.

Navarro, R. (2009). The optical design of the human eye: a

critical review. Journal of Optometry, 2(1):3–18.

Navarro, R., Rozema, J., Emamian, M. H., Hashemi, H.,

and Fotouhi, A. (2019). Average biometry of the

cornea in a large population of iranian school children.

Journal of the Optical Society of America A: Optics,

Image Science, and Vision, 36(4):B85–B92.

erez-Escudero, A., Dorronsoro, C., and Marcos, S. (2010).

Correlation between radius and asphericity in surfaces

ﬁtted by conics. Journal of the Optical Society of

America A, 27(7):1541–1548.

nero, D. P., Nieto, J. C., and Lopez-Miguel, A. (2012).

Characterization of corneal structure in keratoconus.

Journal of Cataract & Refractive Surgery, 38:2167–

2183.

Roffman, J. H. and Menezes, E. V. (1998). Aspheric toric

lens designs. US Patent: US 5796462.

Rosales, M. A., Ju

arez-Aubry, M., L

opez-Olazagasti, E.,

Ibarra, J., and Tepich

ın, E. (2009). Anterior corneal

proﬁle with variable asphericity. Applied Optics,

48(35):6594–6599.

Scholz, K., Messner, A., Eppig, T., Bruenner, H., and Lan-

genbucher, A. (2009). Topography-based assessment

of anterior corneal curvature and asphericity as a func-

tion of age, sex, and refractive status. Journal of

Cataract & Refractive Surgery, 35:1046–1054.

Volatier, J.-B., Duveau, L., and Druart, G. (2020). An ex-

ploration of the freeform two–mirror off–axis solution

space. Journal of Physics: Photonics, 2:1–9.

Wiki-article (2023). Toric lens.

https://en.wikipedia.org/wiki/Toric lens.

PHOTOPTICS 2024 - 12th International Conference on Photonics, Optics and Laser Technology