Improvement in Thermal Resistance of Surface-Emitting Quantum

Cascade Laser by Using a Diamond Submount

Shigeyuki Takagi

, Hirotaka Tanimura

, Tsutomu Kakuno

, Rei Hashimoto

Kei Kaneko

and Shinji Saito

Department of Electrical and Electronics Engineering, School of Engineering, Tokyo University of Technology,

1404-1 Katakura, Hachioji, Tokyo, Japan

Corporate Manufacturing Engineering Center, Toshiba Corporation, 33 Shinisogo, Isogo, Yokohama, Kanagawa, Japan

Keywords: Quantum Cascade Lasers, QCLs, Surface-Emitting QCL, Photonic Crystal, PhC, Static Method,

Structure Function, Thermal Resistance, Thermal Flow Analysis, Diamond Submount.

Abstract: To reduce thermal resistance and improve heat dissipation in surface-emitting quantum cascade lasers

(QCLs), we investigated their structure in which a diamond submount is inserted between a mesa and a CuW

mount. From the results of the thermal flow simulation of three-dimensional models of the QCLs, the thermal

resistance of the QCL without the diamond submount was 8.5 K/Wand that of the QCL with the diamond

submount was 5.2 K/W. From the structure function obtained using the static method, the thermal resistance

of the QCL without the diamond submount was 8.5 K/W and that of the QCL with the diamond submount

was 6.3 K/W. From the measured output range of the QCLs, the measurement output power of the QCL

without the diamond submount was 265 mW and that of the QCL with the diamond submount was 290 mW.

The reduction in thermal resistance and the improvement in laser output were confirmed to be due to the

diamond submount.

1 INTRODUCTION

A quantum cascade laser (QCL) is an n-type

semiconductor laser that emits light in the infrared

region (Faist et al., 1994). Since its emission

wavelength is in the infrared region called the

molecular fingerprint region, the QCL can measure

many gases with high sensitivity. From its merits, it

is expected to be applicable to the detection of trace

substances and distant gases. In the detection of trace

substances, the amount of laser light absorbed is

measured and a long optical path is necessary for laser

light propagation. In the detection of distant gases, a

high-output laser is required because the reflected

light is detected during laser light propagation.

Evans et al. have developed a watt-order laser

oscillation as a high-power laser (Evans et al., 2007).

This laser is an edge-emitting device in which the

direction of the laser light excitation coincides with

that of laser light emission. In contrast, a surface-

emitting QCL that emits laser light from the surface

https://orcid.org/0000-0002-7653-4602

https://orcid.org/0000-0002-1829-6482

in the vertical direction of the device through a

photonic crystal (PhC) was previously developed

(Colombelli et al., 2003). By widening the area of the

excitation part called the mesa, we can expect

improvements in beam quality and heat dissipation.

In our previous study, we developed surface-

emitting QCLs and confirmed single-mode laser

oscillations (Saito et al., 2021, Yoa et al., 2022). In

addition, to evaluate the heat dissipation of these

QCLs, we measured their thermal resistance using the

structure function (Takagi et al., 2019). Furthermore,

we constructed a three-dimensional (3D) thermal

flow simulation model and evaluated the thermal

resistance of these surface-emitting QCLs (Takagi et

al., 2022)

In our current study, we have developed a

prototype of a surface-emitting QCL with a diamond

submount and demonstrated the reduction in thermal

resistance quantitatively. We report these results and

the improvement in laser output.

Takagi, S., Tanimura, H., Kakuno, T., Hashimoto, R., Kaneko, K. and Saito, S.

Improvement in Thermal Resistance of Surface-Emitting Quantum Cascade Laser by Using a Diamond Submount.

DOI: 10.5220/0011777800003408

In Proceedings of the 11th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2023), pages 82-87

ISBN: 978-989-758-632-3; ISSN: 2184-4364

 2023 by SCITEPRESS – Science and Technology Publications, Lda. Under CC license (CC BY-NC-ND 4.0)

2 3D THERMAL FLOW

SIMULATION OF

SURFACE- EMITTING QCL

2.1 Surface-Emitting QCL

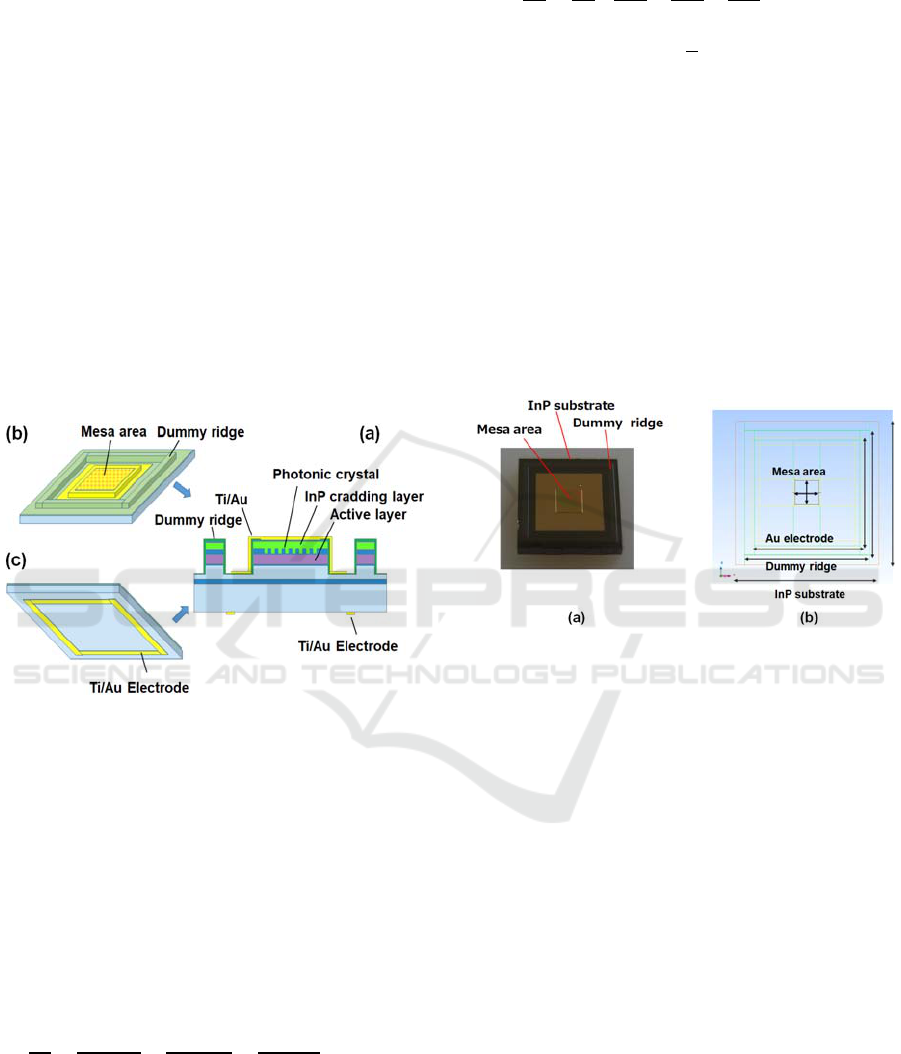
Figure 1 shows the structure of the surface-emitting

QCL in which (a) is a cross-sectional view, (b) is a

view from above, and (c) is a bottom view. A mesa

that emits laser light and a dummy ridge were formed

on an InP substrate with a thickness of 600 µm. In the

mesa area, an InP film was formed on an active layer

(laser excitation portion) that excites a laser, and a

photonic crystal was formed on the InP film. Au

electrodes for current supply was formed on the

opposite side of the InP substrate. A CuW mount was

mounted on the mesa and dummy ridge sides of the

InP substrate, resulting an epi–side–down structure.

Figure 1: Surface-emitting QCL. (a) Cross-sectional view,

(b) top view, and (c) bottom view.

2.2 3D Simulation Model

A simulation model was constructed by inputting the

3D structure and physical property data of the

surface-emitting QCL. The thermal flow analysis

software STEAM (MSC software) was used as the

simulator. The analysis is based on a natural

convection model, in which the laser excitation

portion is overheated and natural convection

occurs (Ho et al., 2008). The equation for gas flow is

𝜕𝜌

𝜕𝑡

∂



𝜌𝑣

𝑥



∂x

∂𝜌𝑣

𝑦



∂



𝜌𝑣

𝑧



∂z

=0,

(1)

where ρ is the density and t is the time. v

, v

, and v

are

the velocities in the x, y, and z directions,

respectively. Moreover, the formula for heat transfer is

𝜕𝑢

𝜕𝑡

𝐾

𝜎𝜌



𝜕



𝑢

𝜕𝑥



𝜕



𝑢

𝜕𝑦



𝜕



𝑢

𝜕𝑧





𝜎

𝐹



𝑥,𝑦,𝑧,𝑡



(2)

where u is the temperature, which is a function of the

position and time, σ is the specific heat, K is the

thermal conductivity, and F is the external heating

value per unit time, which is a function of the position

and time.

Figure 2(a) shows a photograph of the surface-

emitting QCL prototype fabricated on the basis on the

design in Fig. 1. Corresponding to Fig. 1(b), it can be

observed that a mesa and a dummy ridge are formed

on the InP substrate. Figure 2(b) is a simulation model

of the QCL on an InP substrate, showing the outline

of the InP substrate, mesa, dummy ridge, and Au

electrodes on the back surface.

Figure 2: Surface-emitting QCL device: (a) photograph and

(b) simulation model.

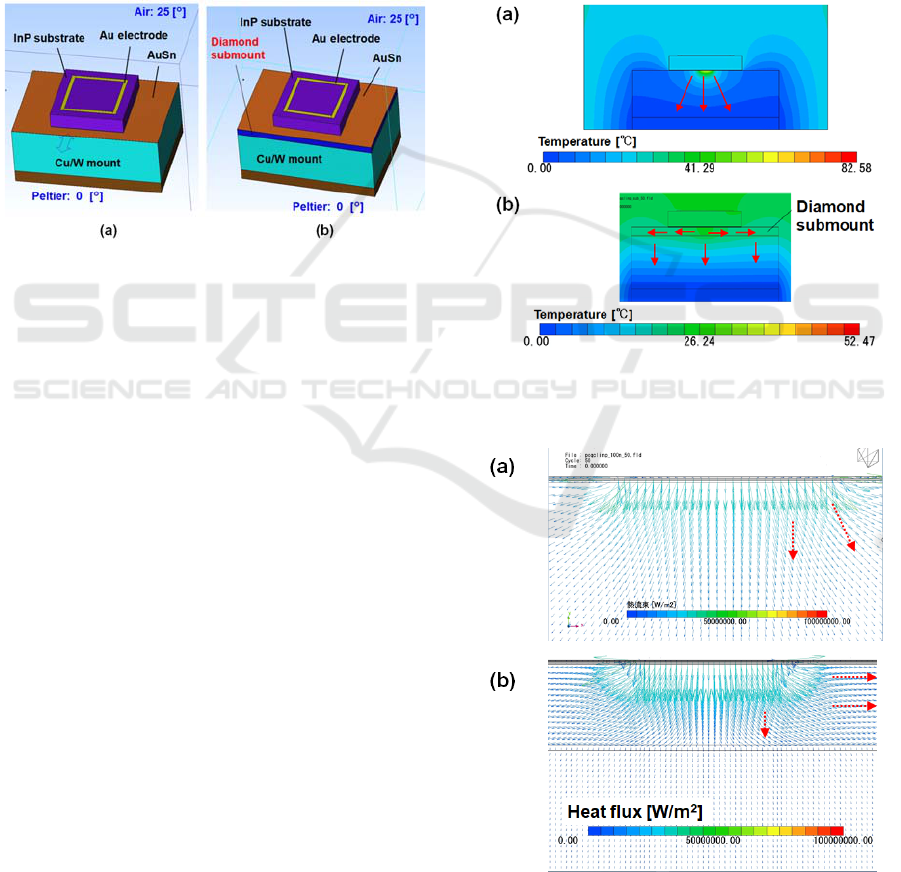
The InP substrate in Fig. 2(a) was mounted on a

CuW mount with an epi–side–down structure. Figure

3(a) shows a 3D model of the QCL on a CuW mount

without a diamond submount. Figure 3(b) shows 3D

model of the QCL mounted with a diamond

submount. Diamond has a high thermal conductivity

and was reported to reduce thermal resistance in light

emitting devices (Bezotosnyi et al., 2014). In the

simulation model, the diamond submount was placed

on the CuW mount and The InP substrate was placed

on the diamond submount with an epi–side–down

structure. The thermal conductivity of the diamond

submount was set at 2000 W/mK.

Other physical property values in this 3D model

are as follows. The thermal conductivities of the CuW

mount, InP, SiO

, Ti, Au, and Cu was 157 W/mK, 68

W/mK, 1.38 W/mK, 21 W/mK, 296 W/mK, and 403

W/mK, respectively. The thermal conductivity of the

Au-buried PhC part was determined from the volume

ratio of photonic crystal and InP cradding. In the laser

pumping part, Al

0.638

0.362

As and Ga

0.331

0.669

As thin

films were alternately laminated. From the

Improvement in Thermal Resistance of Surface-Emitting Quantum Cascade Laser by Using a Diamond Submount

references, the thermal conductivity of

0.638

0.362

As thin film was 10.0 W/mK (Kim et al.,

2002), and that of the Ga

0.331

0.669

As thin film was

5.6 W/mK (Adachi, 1985). From the film thicknesses

of both thin films, the thermal conductivity of the

laminated thin film was calculated to be 7.5 W/mK.

Regarding the temperature boundary conditions, the

temperature of Peltier cooler was fixed at 0°C at the

mount, and the ambient temperature of the surface-

emitting QCL is set at 30°C. The temperature increase

was calculated assuming that the power from the

power source is supplied to the laser pumping

section.

Figure 3: 3D model for thermal flow simulation. (a) QCL

without diamond submount and (b) QCL with diamond

submount.

2.3 Simulation Results

The temperature distribution of the surface-emitting

QCL was calculated with an input power of 10 W to

the laser excitation portion. Figure 4(a) shows the

temperature distribution of the QCL without a

diamond submount, and Fig. 4(b) shows that of the

QCL with the diamond submount. The temperature

distribution in the central cross section of the surface-

emitting QCL is also shown. The temperature of the

Peltier cooler was fixed at 0°C, and that of the CuW

mount was also near 0°C owing to its high thermal

conductivity. The temperature is high in the laser

excitation portion where the power was supplied and

the portion in the mesa around the excitation portion.

The temperature increase at an input power of 10 W

was determined to be 82.63°C in the QCL without a

diamond submount and 52.47 °C in the QCL with a

diamond submount. The temperature increase was

suppressed by using the diamond submount.

In Fig. 4(a), the isotherms under the mesa are

semi-circular, and heat is concentrically transmitted

around the mesa. On the other hand, in Fig.4(b),

owing to the high thermal conductivity of the

diamond submount, the heat spreads horizontally and

is transmitted vertically through the CuW mount. To

investigate the thermal flow in more detail, we

calculated the heat flux of the surface-emitting QCL.

Figure 5(a) shows the distribution of thermal flux

vectors of the QCL without a diamond submount, and

Fig. 5(b) shows that of thermal flux vectors of the

QCL with a submount. In Fig.5(a), the heat is mainly

directed from the mesa, while in Fig 5(b), a horizontal

thermal flux vector is generated in the diamond

submount. From the temperature distribution in Fig.

4 and the thermal flux distribution in Fig. 5, we

consider that heat is transmitted horizontally by the

diamond submount, thereby reducing the thermal

resistance.

Figure 4: Simulation results of temperature distributions.

(a) QCL without diamond submount and (b) QCL with

diamond submount.

Figure 5: Simulation results of heat flux. (a) QCL without

diamond submount and (b) QCLwith diamond submount.

PHOTOPTICS 2023 - 11th International Conference on Photonics, Optics and Laser Technology

Next, we calculated the temperature increase with

respect to the input power. The obtained results are

shown in Fig.6. The temperature increases at 10 W

show a thermal resistance of 8.26 K/W in the QCL

without a diamond submount and a temperature

resistance of 5.25 K/W in the QCL with a diamond

submount. As the input power increases, the

temperature difference between the QCLs with and

without a diamond submount increases. This shows

that the diamond submount is more effective under

high input power operating conditions.

Figure 6: Relationship between input power and mesa

temperature.

3 THERMAL RESISTANCE

MEASUREMENTS OF

SURFACE-EMITTING QCLS

3.1 Static Method and Structure

Function

In this study, a statics method was applied to the

thermal resistance measurements of the surface-

emitting QCLs. In the statics method, after heating

devices, thermal resistance is measured from the

voltage–current characteristics obtained during

cooling. The measurement method has short

measurement time and high reproducibility.

T3Ster (Siemens AG) was used for the

measurement. Since the resistance of a

semiconductor device changes with temperature,

the temperature change is proportional to the

voltage change across the device when a constant

current is allowed to flow. In the statics method,

this voltage change ΔT

[mV] is measured and the

temperature change ΔT

[K] of the device is

obtained as (

Székely, 1997)

∆𝑇



∙∆𝑇



)

where K is a coefficient called the K-factor. A

surface-emitting QCL was set in the thermostat of

T3Ster, and the K-factor was measured by

changing the thermostat temperature from 20℃ to

70℃. As a result, the K-factor was determined as –

0.022772. K/V.

T3Ster was used to measure the structure

function by the statics method. The CuW mount

was cooled to 20°C and heated by supplying

approximately 1.6 W of power to the QCL. After

stopping the heating power supply, the temperature

of the QCL during cooling was measured to obtain

a cooling curve. Assuming that the thermal

resistance and thermal capacity of the elements

constituting the QCL are R

and C

, respectively,

the time constant τ during cooling is expressed as

𝜏=𝐶



∙𝑅



)

3.2 Measurement Results

Surface-emitting QCLs of the same lot were

mounted with and without a diamond submount.

Figures 7(a) and (b) show the QCL mounted

without a diamond submount and the QCL

mounted with a diamond submount, respectively.

The CuW mount and surface-emitting QCL were

soldered to the diamond submount with indium.

Figure 7: Photographs of the mounted surface-emitting

QCLs: (a) without and (b) with diamond submount.

Figures 8(a) and (b) show the cooling curves of

the QCLs without diamond submount and with the

diamond submount measured by the static method,

respectively. Figures 9(a) and (b) show the

structure functions of the QCLs without and with

the diamond submount obtained from the cooling

curves shown in Fig. 8, respectively. In the flat area

above 8.5 K/W in (a), the thermal resistance value

fluctuates when the mounting method of the

surface-emitting QCL is changed during

measurement. Therefore, the thermal resistance

Improvement in Thermal Resistance of Surface-Emitting Quantum Cascade Laser by Using a Diamond Submount

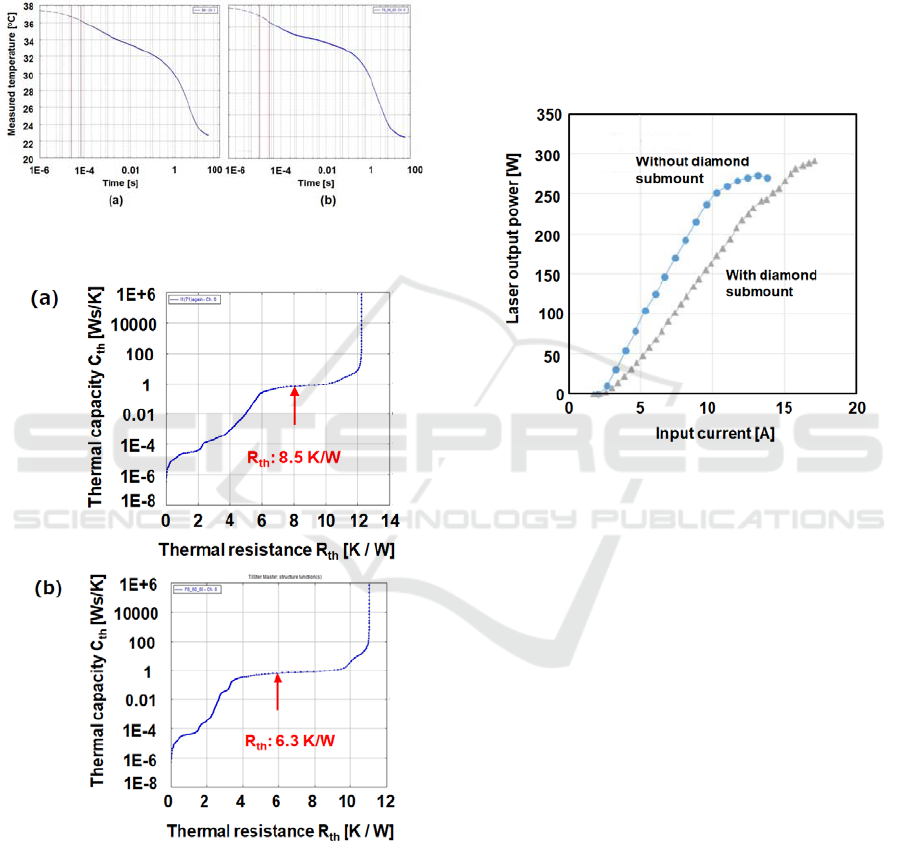
over of the flat region was considered to be the

thermal resistance between the surface-emitting

QCL and the T3Ster cooler. We estimated a typical

value of 8.5 K/W for the total thermal resistance of

surface-emitting QCLs. Similarly, the thermal

resistance of the QCL in (b) was estimated to be 6.3

K/W.

Figure 8: Cooling curves of QCLs (a) without and (b) with

diamond submount.

Figure 9: Structure functions of QCLs (a) without and with

diamond submount (b).

4 LASER OSCILLATION

To investigate the effect of the diamond submount,

the laser power was measured. The QCL operating

conditions were a frequency of 100 KHz, a duty of

1%, and a cooling temperature of 77 K. Figure 10

shows the measurement results. Although increases

with the supply current, the laser output stops

increasing at around 12 A for the QCL without a

diamond submount. In contrast, the QCL with a

diamond submount increases in the output even at a

current of 17 A. The maximum output power within

the measured range was determined 265 mW for the

QCL without a diamond submount and 290 mW for

the QCL with a diamond submount. This indicates

that the diamond submount improved the heat

dissipation and output of the QCL.

Figure 10: Output powers of QCLs without and with

diamond submount.

5 CONCLUSIONS

To reduce thermal resistance and improve heat

dissipation in surface-emitting QCLs, we investigated

their structure in which a diamond submount is

inserted between a mesa and a CuW mount. From the

thermal flow simulation of 3D models of the QCLs,

the thermal resistance of the QCL without the

diamond submunt was 8.5 K/W and that of the QCL

with the diamond submount was 5.2 K/W. From the

structure function obtained using the static method,

the thermal resistance of the QCL without the

diamond submount was 8.5 K/W and that of the QCL

with the diamond submount was 6.3 K/W. The

simulation results and structure function of the QCL

with the diamond submount show that the reduction

in thermal resistance is caused by the diamond

submount. From the measured output range of the

QCLs, the maximum output power of the QCL

without the diamond submounit was 265 mW and that

of the QCL with the diamond submount was 290 mW.

The reduction in thermal resistance and the

PHOTOPTICS 2023 - 11th International Conference on Photonics, Optics and Laser Technology

improvement in laser output were confirmed to be

due to the diamond submount.

ACKNOWLEDGEMENTS

This work was supported by Innovative Science and

Technology Initiative for Security (Grant Number

JPJ004596), ATLA, Japan.

REFERENCES

Faist, J., Capasso, F., Sivco, D. L., Sirtori, C., Hutchinson,

A., & Cho, A. Y. (1994). Quantum cascade laser.

Science, 264, 553–556.

Evans, A., Darvish, S. R., Slivken, S., Nguyen, J., Bai, Y.,

& Razeghi, M. (2007). Buried heterostructure quantum

cascade lasers with high continuous-wave wall plug

efficiency. Appl. Phys. Lett., 91, 071101-1–3.

Colombelli, R., Srinivasan, K., Troccoli, M., Painter, O.,

Gmachl, C. F., Tennant, D. F., Sergent, A. M., Sivco,

D. L., Cho, A. Y., & Capasso, F. (2003). Quantum

cascade surface-emitting photonic crystal laser.

Science, 302, 1374–1377.

Saito, S., Hashimoto, R., Kaneko, K., Kakuno, T., Yao, Y.,

Ikeda, N., Sugimoto, Y., Mano, Y., Kuroda, T.,

Tanimura, H., Takagi, S., & Sakoda, K. (2021). Design

and fabrication of photonic crystal resonators for

single-mode and vertical surface emission from strain-

compensated quantum cascade lasers operating at 4.32

μm. Appl. Phys. Express, 14, 102003-1–5.

Yao, Y., Ikeda, N., Chalimah, S., Kuroda, T., Sugimoto, Y.,

Mano, T., Koyama, H., Hashimoto, R., Kaneko, K.,

Kakuno, T., Ookuma, S., Togawa, T., Ohno, H., Saito,

S., Takahashi, H., Tanimura, H., Takagi, S., & Sakoda,

K. (2022). Improved power and far-field pattern of

surface-emitting quantum cascade lasers with strain

compensation to operate at 4.3 μm”, Jpn. J. Appl. Phys.

61, 052001-1–8.

Takagi, S., Tanimura, H., Kakuno, T., Hashimoto, & R.,

Saito, S. (2019). Thermal analysis and heat dissipation

improvement for quantum cascade lasers through

experiments, simulations, and structure function. Jpn.

J. Appl. Phys., 58, 091008-1–6.

Takagi, S., Tanimura, H., Kakuno, T., Hashimoto, R., Saito,

S. (2022). Improvement of thermal resistance of

surface-emitting quantum cascade laser using structural

function and 3D thermal flow simulation. 10

International Conference on Photonics, Optics, and

Laser Technology, 128–132.

Ho, C. J., Chen, M. W., & Li, Z. W. (2008). Numerical

simulation of natural convection of nanofluid in a

square enclosure: Effects due to uncertainties of

viscosity and thermal conductivity, Int. J. Heat Mass

Transfer, 51, 4506–4516.

Bezotosnyi, V. V., Krokhin, O. N., Oleshchenko, V. N.,

Pevtsov, V.A., Popov, Y. M., & Cheshev, E. A. (2014).

Thermal modelling of high-power laser diodes mounted

using various types of submount. IEEE J. Quantum

Electron., 44, 899–902.

Kim, Y. M., Rodwell, M. J. W., & Gossard, A. C. (2002).

Thermal characteristics of InP, InAlAs, and AlGaAsSb

metamorphic buffer layers used in In

0.52

0.48

0.53

0.47

As heterojunction bipolar transistors grown

on GaAs substrates. J. Electron. Mater., 31, 196–199.

Adachi, S. (1985). GaAs, AlAs, and AlxGa1−xAs: Material

parameters for use in research and device applications.

J. Appl. Phys., 58, R1–R29.

Székely, V. (1997). A new evaluation method of thermal

transient measurement results. Microelectron. J., 28,

277–292.

Improvement in Thermal Resistance of Surface-Emitting Quantum Cascade Laser by Using a Diamond Submount