Generalized Poincaré Plots Analysis of Cardiac Interbeat Intervals in

Heart Failure

Mirjana M. Platiša

, Nikola N. Radovanović

, Aleksandar Kalauzi

and Siniša Pavlović

University of Belgrade, Faculty of Medicine, Institute of Biophysics, 11129 Belgrade, Serbia

University of Belgrade, University Clinical Center of Serbia, Pacemaker Center, 11000 Belgrade, Serbia

University of Belgrade, Institute for Multidisciplinary Research, Department for Life Sciences, 11000 Belgrade, Serbia

Keywords: Generalized Poincaré Plots Analysis, Lagged Poincaré Plots Analysis, Heart Rate Variability, Heart Failure.

Abstract: In this work we applied generalized Poincaré plots (gPp) analysis of interbeat intervals in patients with heart

failure. More, we compared gPp with its nearest analogy methods based on existing extended Poincaré plots

techniques. Obtained results showed advantages of gPp method over usually used distanced (lagged) Poincaré

plots analysis. Only gPp has the potential of three-dimensional visualization of results with quantification of

new multiscaling parameters. It is comparable with other methods only in two-dimensional planes where all

methods showed a strong negative correlation between patterns of Pearson correlation coefficients and

patterns of the SD1/SD2 ratio over the whole range of Pp orders (lags). These results could be used as the

basis for further research in new standardization of multiscaling methods in heart rhythm analysis where it is

important to follow the pattern of regulatory mechanisms dynamics which is related to the duration of RR

intervals.

1 INTRODUCTION

Analysis of heart rate variability (HRV) is used to

extract important information about the interaction

between the cardiovascular system and the nervous

system. Usually, linear and nonlinear methods of

HRV analysis which revealed the structure and

properties of interbeat interval series are applied with

several main issues. In medicine, dominantly, through

the application as diagnosis of autonomic dysfunction

in various disease and prognostic information

contained in HRV as a reflection of the activity of the

autonomic nervous system and mechanical heart

function. Additionally, other multidisciplinary goals

were to develop new methods which may better

describe dynamics of the cardiovascular system and

help to reveal and understand some important

information about regulatory mechanisms and their

changes in different functions of the autonomic

nervous.

https://orcid.org/0000-0002-0915-2823

https://orcid.org/0000-0002-6545-2230

https://orcid.org/0000-0003-4833-0757

https://orcid.org/0000-0002-1395-4407

The standardized Poincaré plot (Pp) is a

representation of a time series into a phase space with

delay or lag of one point i.e. in the case of HRV

analysis each RR interval is plotted as a function of

the previous RR interval. First of all, it presents useful

visual information and it is a part of standardized

methods of HRV analysis. There is also a quantitative

analysis of the Poincaré scattergram by SD1 –

variance of RR intervals in a short scale, SD2 –

variance in a long term scale and their ratio SD1/SD2.

Moreover, the full potential of this approach is

searched through the extension of Pp analysis by

autocovariance concept at higher lags (Lerma et al,

2003, Thakre and Smith, 2006). Standardized

Poincaré plot analysis is one method of HRV analysis

derived from nonlinear dynamics analysis. However,

some indices derived from this approach are strong

correlates to the linear HRV measures derived from

spectral analysis (Contreras et al, 2007).

Recently we proposed a new, generalized Pp

analysis method and applied it to ECG recordings of

Platiša, M., Radovanovi

c, N., Kalauzi, A. and Pavlovi

c, S.

Generalized Poincaré Plots Analysis of Cardiac Interbeat Intervals in Heart Failure.

DOI: 10.5220/0010912400003123

In Proceedings of the 15th International Joint Conference on Biomedical Engineering Systems and Technologies (BIOSTEC 2022) - Volume 4: BIOSIGNALS, pages 251-256

ISBN: 978-989-758-552-4; ISSN: 2184-4305

251

patients with permanent atrial fibrillation (Platiša et

al, 2016), and to ECG recordings of three different

physiological conditions in young healthy trained and

untrained subjects (Platiša et al, 2019b). Contrary to

other lagged (distanced) Poincare plots methods, this

unique method instead RR interval used R peak in

ECG recordings as a referent point for further

analysis. This approach allows us to follow RR

intervals and their duration on both sides of chosen R

peak and calculate correlations between symmetrical

distanced duration of RR intervals and moreover

asymmetrically distanced durations of RR intervals.

There were a lot of results obtained with this gPp

analysis and only part of them indicate possible

interpretation which could be associated with well

known physiological mechanisms, primarily directly

related to the parasympathetic cardiac control.

The aim of this study is twofold. The first one was

to apply gPp method to ECG recordings of heart

failure patients and the second one to compare this

method with the most approximate variants of

extended Poincare plots analyses.

2 METHOD

2.1 Subjects

We included 63 patients with symptomatic heart

failure (HF) and reduced left ventricular ejection

fraction (LVEF < 35%) who had an indication for an

implantable cardioverter-defibrillator or cardiac

resynchronization therapy device implantation.

Patients were divided into two groups depending on

whether they were in sinus rhythm (HFSin) or with

permanent atrial fibrillation (HFAF). In the HFSin

group, we analyzed 30 heart failure patients (5

women) aged 57.9 ± 7.5 years; while in the (HFAF)

33 heart failure patients (3 women) aged 68.0 ± 7.6

years. The control group consists of 30 healthy

middle-aged subjects (15 women) aged 46.3 ± 8.2

years, all were nonsmokers without any history of

disease. All participants were instructed to avoid

physical activity starting the day prior to

measurement and to not eat or drink on the day of the

examination.

2.2 Experimental Data

Measurements were done in the Pacemaker Centre of

the University Clinical Centre Serbia and in the

Laboratory for Biosignals, Institute of Biophysics,

Faculty of Medicine. Ethics Committee of the Faculty

of Medicine, University of Belgrade approved this

study. All subjects gave written informed consent in

accordance with the Declaration of Helsinki.

Experiments were done in the morning between 8:00

and 11:00 a.m.

ECG recordings were obtained from subjects in

the supine position and with spontaneous breathing,

20 minutes in duration (without moving and verbal

communication). The ECG was acquired with

sampling frequency of 1 kHz by Biopac MP100

system with AcqKnowledge 3.9.1. software

(BIOPAC System, Inc., Santa Barbara, CA, USA).

We analysed RR intervals extracted from 20

minutes of supine relaxing ECG recordings.

Sequences of approximately 1200 RR intervals

extracted from ECG data were obtained using

OriginPro 8.6 (OriginLab Corporation, USA).

2.3 Standardized Poincaré Plot

A typical HRV Poincaré plot represents scatter graph

as the function (RR)

i+1

= f((RR)

). The two standard

parameters SD1 and SD2, called the Poincaré plot

descriptors, describe the distribution of points around

two diagonals. It is accepted that SD1 describes

instant heartbeat intervals variability and quantifies

short-term HRV, while SD2 quantifies long-term

HRV (Platiša et al, 2019a).

2.4 Generalized Poincaré Plot Analysis

Our recently proposed method is generalized form of

Poincaré plot analysis where we track dependence

and correlation between the duration of j preceding

and k next RR intervals by varying j and k around

chosen R peak in ECG (Platiša et al, 2016, Platiša et

al, 2019b).

Figure 1: Simplified example of chosen sliding R peak in

ECG recording and the duration of k preceding and k next

RR interval.

Both quantities were calculated by simply adding the

durations of the corresponding intervals around a

BIOSIGNALS 2022 - 15th International Conference on Bio-inspired Systems and Signal Processing

252

chosen R wave which was moving along the ECG

signal (Figure 1).

For an ECG signal with a total

number of N RR intervals, only N – j – k points could

be drawn. For results obtained with specific values of

j and k, i.e. for a pair of number of intervals, (j,k), we

propose the term "order of the gPp". In cases when

symmetric (j = k) gPp were calculated, for previous k

and following k intervals in duration, integral

dynamics of interbeat intervals appeared as specific

trajectories in relaxed healthy subjects (Platiša et al.,

2016, Platiša et al., 2019b).

Moreover, in cases when asymmetric (j ≠ k) gPp

were calculated the relationship between previous j

and following k summed intervals give as additional

information about dynamics of cardiac control

mechanisms. In this work we quantified relationship

between summed durations of j previous and k

following consecutive RR intervals by Pearson

correlation coefficients of matrices for j, k = 1, …, 50

intervals, which we brieﬂy denoted as r(j, k). In order

to quantify asymmetry of matrices, we introduce a

normalized asymmetry index (NAI), which for a m×n

type matrix is defined as













kjrjkr

NAI

),(),(

(1)

where r(j,k) represents matrix element, while









kjr

),(

(2)

Another interesting property of these Pearson's

matrices was the appearance and positions of local

maxima, since each local maximum of correlation

could potentially signify a temporal range in which a

neurocardiac regulatory mechanism is operating.

All analyses were performed using our original

programs developed within MATLAB (MathWorks

Inc., Natick, MA01760-2098, United States).

2.5 Distanced Generalized Poincaré

Plots Analysis

In order to compare our gPp method with the

published methods of extended Poincaré plot analysis

we used two approaches. The first one is the nearest

analogy to gPp which we called distanced generalized

Poincaré plots (dgPp) method. In this method we

examined relations between individual RR intervals,

the k preceding and the k following RR interval

around chosen R peak in ECG. During calculation we

noticed one important limitation of this approach.

When we increase distance (Pp order) by one, RR

intervals are distanced away for two intervals. Hence,

only even RR intervals are analysed, and odd RR

intervals are omitted.

2.6 Distanced Poincaré Plots Analysis

This approach actually represents lagged (extended)

standardized Poinacre plot analysis which can be

found in published literature. Instead of arbitrary

chosen R peak in ECG signal, in this approach we

started calculation with fixed, the first RR interval in

time series of RR intervals. For k = 1, we track two

neighbor RR intervals in the whole series, for k = 2

we track RR intervals distanced by one RR interval,

etc.

2.7 Statistics

Mann-Whitney U test was used to compare indexes

of asymmetry between the group of healthy subjects

and two groups of HF patients.

The data of NAI are

given as mean values ± standard errors. Statistical

analyses were performed using the software package

SPSS Statistics (version 17.0, SPSS Inc, USA). A

value of p < 0.05 was considered significant.

3 RESULTS

3.1 Generalized Poincaré Plots

Analysis in Heart Failure

In order to present the major difference between

standardized Pp and generalized Pps analysis we

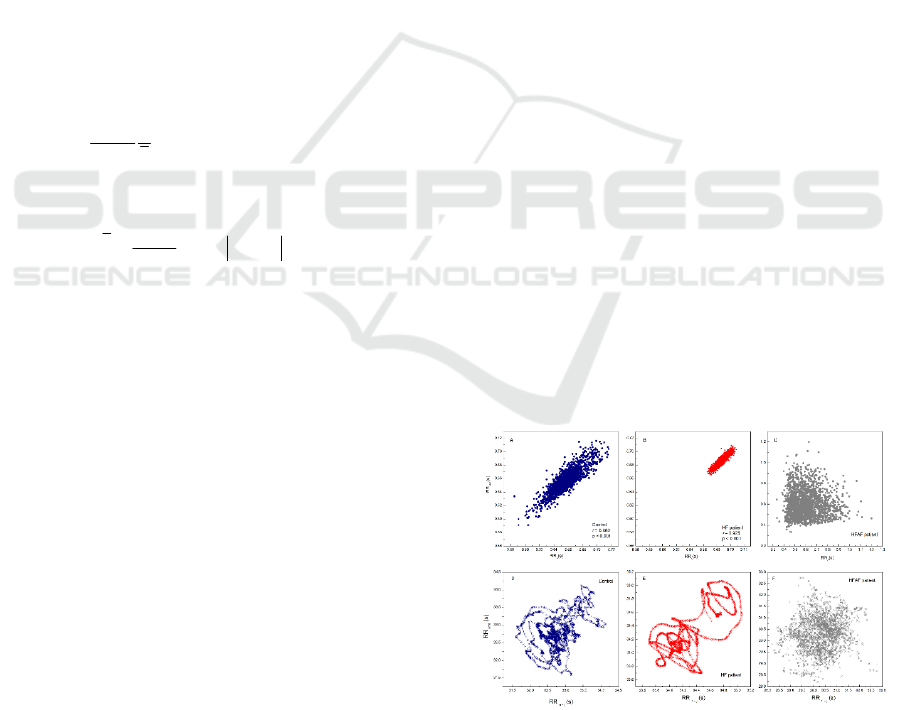
show in Figure 2 examples of standardized Poincaré

plot and generalized Poincaré plots of k order in one

healthy control subject and two heart failure patients

Figure 2: Examples of standardized Pp (top) and gPp for k

= 50 (bottom) of RR intervals for one control subject (blue)

and two subjects with heart failure (with sinus rhythm in red

and with atrial fibrillation in grey).

Generalized Poincaré Plots Analysis of Cardiac Interbeat Intervals in Heart Failure

253

(one with sinus rhythm and one with atrial

fibrillation). In the gPp analysis we denote by k the

order of gPp which means successive sums of RR

intervals, in this paper up to 50.

The presence of hanks in gPp method is one of the

first advantages of this approach. Considering these

with our previous findings, we can conclude that

hanks indicate complex dynamical processes in

autonomic cardiac control dominantly related to

transition pathways of parasympathetic cardiac

control. It is interesting that hanks are also present in

heart failure patient with sinus rhythm although

variability of this time series is reduced compared

with Pp (variability of time series) in healthy control

subject (Figure 2). This finding and finding in heart

failure subject with atrial fibrillation patient where

autonomic cardiac control via sinus node ceased to

exist suggested that non-existence of hanks indicated

absence of parasympathetic cardiac control and that

their existence was not related to heart rate variability.

Figure 3: Examples of matrices of Pearson correlation

coefficients for (j, k ≤ 50) in a one control subject and two

heart failure patients.

Further, we calculated matrix of Pearson

correlation coefficients for (j, k = 50) and present

representative examples in Figure 3. The area of

correlation coefficients between summed RR

intervals for heart failure patient with sinus rhythm

was positioned on the top, above the area of

correlation coefficients for healthy subject and the

area of correlation coefficients which belongs to heart

failure patient with atrial fibrillation.

This position which had mild decrease with the

highest order of Pp indicated strongly correlated

summed RR intervals up to the 50

order of Pp. On

the other hand, in control subject summed RR

intervals were strongly correlated only in the small

range of few first orders of Pp, while in HFAF patient

Pearson correlation coefficients were reduced over

Figure 4: Pooled local maxima of Pearson’s correlation

coefficients matrices projected on the (j, k) plane in the

group of control subjects (A), in the group of HFSin patients

(B) and in the group of HFAF patients (C).

Figure 5: Distribution of pooled maxima of Pearson’s

correlation coefficients matrices in the group of healthy

subjects (blue), in the group of HFSin patients (red) and in

the group of HFAF patients (grey) plotted in 3D.

the all area. Pooled local maxima of Pearson

correlation matrix in each group were determined and

they are presented in Figure 4 and Figure 5. More, we

determined normalized index of asymmetry (NAI)

and compared their mean values between the groups.

There were statistically significant difference only

between the group of control and the HFSin group

(Figure 6).

BIOSIGNALS 2022 - 15th International Conference on Bio-inspired Systems and Signal Processing

254

Figure 6: Normalized asymmetry index (NAI). Data are

presented as mean with standard errors.

3.2 Comparisons of gPp with Other

Methods

Unfortunately, nature of the other approaches in Pp

analysis is limited and results were not comparable in

three-dimensional space. Hence, we continued

further analysis in 2D plots only for symmetric (j = k)

diagonals elements of Pearson correlation

coefficients matrices and the SD1/SD2 ratio for all

gPp orders (k = 1 ,…, 50).

Figure 7: Figure shows dependencies of averaged Pearson

correlation coefficient and averaged the ratio SD1/SD2

parameters for all three approaches in the group of control

healthy subjects and the HFSin and the HFAF group.

In results obatined from all methods we found

strong negative correlation between patterns of the

ratio SD1/SD2 and patterns of the Pearson correlation

coefficients over the whole range of Pp orders (Figure

7).

4 DISCUSSION

In this paper, we describe results of applied recently

proposed gPp analysis of RR intervals in heart failure

patients and control subjects. Consistent with

findings in our previous studies, we showed that gPp

analysis can also differentiate heart failure patients

with sinus rhythm and with atrial fibrillation. It is well

known that reduced heart rate variability is one of the

major properties of sinus rhythm in heart failure

patients. Besides this property, approved with

standardized Poincare plot, we showed that the

existence of hanks was not influenced by reduced

HRV in the group of HFSin patients. In our previous

papers we found that activities of parasympathetic

cardiovascular control were described by hanks

(Platiša et al, 2016, Platiša et al, 2019b).

Further, we obtained, from gPp Pearson

coefficient matrix, strong correlations between

summed RR intervals in HFSin patients over all

orders (k = j = 50), while in healthy subjects strong

correlations are present only for few first orders of

summed RR intervals. Beside estimated strength of

correlations i.e. Pearson correlation coefficients we

determined local maxima of Pearson correlation

coefficients and calculated normalized index of

asymmetry (NAI). Positions of local maxima in the

HFSin patients group were more distanced from the

central diagonal while in healthy subjects they are

close to this diagonal and there is much more number

of maxima on the diagonal. In the group of HFAF

patients, we had almost random distribution of local

maxima with very low values of Pearson correlation

coefficients. Similar result we obtained in our

previously published paper (Platiša et al, 2016) where

we analyzed data from patients with permanent atrial

fibrillation but without heart failure. It is obviously

that impact of heart failure in cases with atrial

fibrillation on the gPp analysis results is very low or

almost neglectable.

At this moment, we didn’t recognize any

particular physiological mechanisms related to the

maxima on areas of Pearson correlation coefficients.

They may be related to time scales over which

physiological mechanisms operate via the duration of

summed RR intervals. As additional information, we

captured the integral information from positions of

pooled maxima in each group of subjects and

quantified it by NAI. In both groups of heart failure

patients NAI is more negative than in the control

group but there is a statistically significant difference

only between the HFSin group and the control group.

Furthermore, we show that independently of

Poincaré plot analysis approach, over all orders of Pp

(or of lags), a strong relationship between the pattern

of Pearson coefficients and the pattern of the

SD1/SD2 ratio exited (like an inverted picture in the

mirror).

Generalized Poincaré Plots Analysis of Cardiac Interbeat Intervals in Heart Failure

255

Averaged Pearson correlation coefficients

calculated by gPp just for diametrical, symmetric

cases (j = k), changed with gPp orders and they were

lowest for the HFAF group. It is very interesting that

changes of Pearson correlation coefficients with k

were noticeable up to the 13

order of gPp analysis in

all analyzed groups. Similarly, inverted changes can

be seen in dependence of the SD1/SD2 ratio on the

gPp order k. In the control group there is the maxima

for k = 1 and minimal correlations approximately

around 3th or 4th order, while in HFSin group

maxima is at k = 3, and the value of coefficients

between the group are different up to 10

gPp order.

These maxima and minima positions probably

revealed times at which operated dominant regulatory

cardiac control mechanisms.

In dgPp analysis, a real analogy technique to the

gPp, we obtained different dependencies of Pearson

coefficients on the k in analyzed groups. As expected,

Pearson coefficients in the HFAF group were close to

zero and the SD1/SD2 ratio around 1. In the HFSin

group, Pearson coefficients monotonically decreased

with k, while in control subjects there were two

maxima in dependence of Pearson coefficients of k (at

k =1 and at k ≈ 5). In simple lagged Pp analysis, which

we called dPp, we obtained similar dependencies of

Pearson coefficients and the SD1/SD2 on lag k as in

dgPp, but they were stretched because in calculation

entered all RR intervals.

5 CONCLUSIONS

In summary, we showed advantages of generalized

Pp analysis which takes referent R peak in ECG

recording instead of referent RR interval in analysis

of heart rhythm. This approach for symmetrical cases

(j = k) revealed interesting patterns of integrated

cardiac control which were not related to the absolute

value of heart rate variability, well-known measure of

autonomic cardiac control. In the extended part of

gPp analysis, when asymmetrically cases (j ≠ k) were

involved, areas of Pearson correlation coefficients

matrices were obtained whose physiological

background needs to be discovered in further work.

REFERENCES

Lerma, C., Infante, O., Pérez-Grovas, H., & José, M. V.

(2003). Poincaré plot indexes of heart rate variability

capture dynamic adaptations after haemodialysis in

chronic renal failure patients. Clinical physiology and

functional imaging, 23(2), 72–80.

Thakre, T. P., & Smith, M. L. (2006). Loss of lag-response

curvilinearity of indices of heart rate variability in

congestive heart failure. BMC cardiovascular

disorders, 6, 27. https://doi.org/10.1186/1471-2261-6-

27.

Contreras, P., Canetti, R., & Migliaro, E. R. (2007).

Correlations between frequency-domain HRV indices

and lagged Poincaré plot width in healthy and diabetic

subjects. Physiological measurement, 28(1), 85–94.

Platiša, M. M., Bojić, T., Pavlović, S. U., Radovanović, N.

N., & Kalauzi, A. (2016). Generalized Poincaré Plots-

A New Method for Evaluation of Regimes in Cardiac

Neural Control in Atrial Fibrillation and Healthy

Subjects. Frontiers in neuroscience, 10, 38.

Platiša, M. M., Radovanović, N. N., Kalauzi, A.,

Milašinović, G., & Pavlović, S. U. (2019a).

Differentiation of Heart Failure Patients by the Ratio of

the Scaling Exponents of Cardiac Interbeat Intervals.

Frontiers in physiology, 10, 570.

Platiša, M. M., Bojić, T., Mazić, S., & Kalauzi, A. (2019b).

Generalized Poincaré plots analysis of heart period

dynamics in different physiological conditions: Trained

vs. untrained men. PloS One, 14(7), e0219281.

Goshvarpour, A., & Goshvarpour, A. (2020). Asymmetry

of lagged Poincare plot in heart rate signals during

meditation. Journal of traditional and complementary

medicine, 11(1), 16–21.

BIOSIGNALS 2022 - 15th International Conference on Bio-inspired Systems and Signal Processing

256