Local Antimagic Vertex Coloring of Wheel Graph and Helm Graph

F. F. Hadiputra

, D. R. Silaban

and T. K. Maryati

Department of Mathematics, Universitas Indonesia, Depok, Indonesia

Department of Mathematics Education, UIN Syarif Hidayatullah Jakarta, Indonesia

Keywords: Local Antimagic, Vertex Coloring, Wheel Graph, Helm Graph.

Abstract: Let 𝜒



𝐺



be a chromatic number of vertex coloring of a graph G. A bijection 𝑓:𝐸→



1,2,3,…,

𝐸



𝐺

|



called local antimagic vertex coloring if for any adjacent vertices do not share the same weight, where the

weight of a vertex in 𝐺 is the sum of the label of edges incident to it. We denote the minimum number of

distinct weight of vertices in 𝐺 so that the graph 𝐺 admits a local antimagic vertex coloring as 𝜒





𝐺



. In

this study, we established the missing value of 𝜒



for a case in wheel graph and 𝜒



for helm graph.

1 INTRODUCTION

Suppose 𝐺



𝑉,𝐸



be a connected simple graph such

that 𝑣,𝑢∈ 𝑉



𝐺



. We define local antimagic vertex

coloring of 𝐺 as a bijection 𝑓:𝐸→



1,2,3,…,

𝐸



𝐺

|



such that for any adjacent vertices

𝑣 and 𝑢, 𝑤



𝑣



𝑤



𝑢



, which 𝑤



𝑣





∑

𝑓



𝑒



∈







for every edge 𝑒 incident to 𝑣. We are able to

distinguish weights of vertices by assigning distinct

colors for every distinct weights. Using a well-

known notation, 𝜒



𝐺



denoted as the chromatic

number of 𝐺. The local antimagic vertex chromatic

number 𝜒





𝐺



is the minimum number of colors for

vertices taken over all colorings induced by local

antimagic vertex coloring of 𝐺. A remark written by

Arumugam et al. (2017) tells us that for any graph

𝐺, 𝜒





𝐺



𝜒



𝐺



Hartsfield & Ringel (1990) introduced the term

of antimagic labeling of a graph. We can see many

variations of this antimagic labeling. One of many

variations is a concept of local antimagic vertex

coloring introduced by Arumugam et al. (2017).

They also give the exact values for 𝜒



for wheel 𝑊



when 𝑛≢0



mod4



. For 𝑛≡0



mod4



, they found

only the interval.

Arumugam et al. (2018) found the exact value

𝜒





𝐺



for some corona product graphs. Nazula et

al. (2018) established the exact value of 𝜒





𝐺



for

certain unicyclic graphs, which are kite graphs and

cycle graphs with two neighbour pendants. Lau et al.

(2018) showed further results of local antimagic

vertex coloring for some graphs and established a

sharp lower bound for graphs which we use in our

proof. Haslegrave (2018) proved a conjecture

proposed by Arumugam et al. whether any

connected graph other than 𝐾



admits a local

antimagic vertex coloring, by using probabilistic

method.

In this paper, we study local antimagic vertex

coloring for wheel graphs and helm graphs. We

establish an exact value of 𝜒



for a case in wheel

graph, that has not been proved yet by Arumugam et

al. Also, we have exact values of 𝜒



for helm

graphs. Silaban et al. (2009) gave an efficient way of

labeling by defining some conditional function

which we use much in our paper.

2 SUPPLEMENTARY

PROPERTIES

For convenience, we would like to introduce some

simpler notations that we use in this paper. Firstly,

we denote 𝑖∈



𝑎,𝑏



as 𝑖 being an integer greater or

equal to 𝑎, while lower or equal to 𝑏. Next, we add

additional index of 𝑒 or 𝑜, as in 𝑖∈



𝑎,𝑏





that has

additional information of 𝑖 an even integer, while

using 𝑜 simply means 𝑖 an odd integer.

Silaban et al. (2009) introduced a function which

checks a condition of certain value and returns

according to whether the condition is satisfied. One

of the example is the odd



𝑥



function which defined

as follows

Hadiputra, F., Silaban, D. and Maryati, T.

Local Antimagic Vertex Coloring of Wheel Graph and Helm Graph.

DOI: 10.5220/0010138400002775

In Proceedings of the 1st International MIPAnet Conference on Science and Mathematics (IMC-SciMath 2019), pages 185-189

ISBN: 978-989-758-556-2

185

odd



𝑖





1, if 𝑖≡1



mod2



0, otherwise.

We will use this convenient function in our

proofs. Other than that, we would like to introduce

another function called modulo congruency check.

Modulo congruency check 𝑚



𝑥,𝑡



is a function that

values to 1 if 𝑥 is equivalent 𝑡 by mod 4, while

otherwise 0. Formally, we write as follows

𝑚



𝑖,𝑡





1, if 𝑖≡𝑡



mod4



0, otherwise.

We use a definition of the wheel graph 𝑊



order 𝑛1 with the vertex set

𝑉



𝑊









𝑐,𝑣



|𝑖∈



1,𝑛



and the edge set

𝐸



𝑊









𝑣



𝑣



,𝑐𝑣



,𝑣



𝑣



,𝑐𝑣



|𝑖∈



1,𝑛 1



Arumugam et al. (2017) proved the exact value

of 𝜒



in many cases of wheel graphs as follows

Theorem 1 (Arumugam et al., 2017). For the wheel

𝑊



of order 𝑛1, we have

𝜒





𝑊







4, if 𝑛≡1,3



mod4



3, if 𝑛≡2



mod4



For 𝑛≡0



mod4



, the authors found only the

interval 3𝜒





𝑊





5. They also found a sharp

lower bounds for arbitrary tree graph. Lau et al.

(2018) generalizes this theorem as follows

Theorem 2 (Lau et al., 2018). Let 𝐺 be a graph

having 𝑘 pendants. If 𝐺 is not 𝐾



, then 𝜒





𝐺





𝑘1 and the bound is sharp.

The preceding theorem is useful for finding a

lower bound of 𝜒





𝐻





. We continue this reasoning

to have sharp lower bounds for this particular helm

graphs.

3 MAIN RESULTS

We start to establish our main theorem.

Theorem 3. Let 𝑛≡0



mod4



. Then 𝜒





𝑊





3.

Proof. It is known that

𝜒



𝑊







4, if 𝑛 is odd,

3, if 𝑛 is even.

(1)

Therefore, for 𝑛≡0



mod4



, 𝜒





𝑊





3. To

show 𝜒





𝑊





3 for 𝑛≡0



mod4



, we will

define 𝑓:𝐸



𝑊





→



1,2,3,…,

𝐸



𝑊



|



that admits

local antimagic vertex coloring of 𝑊



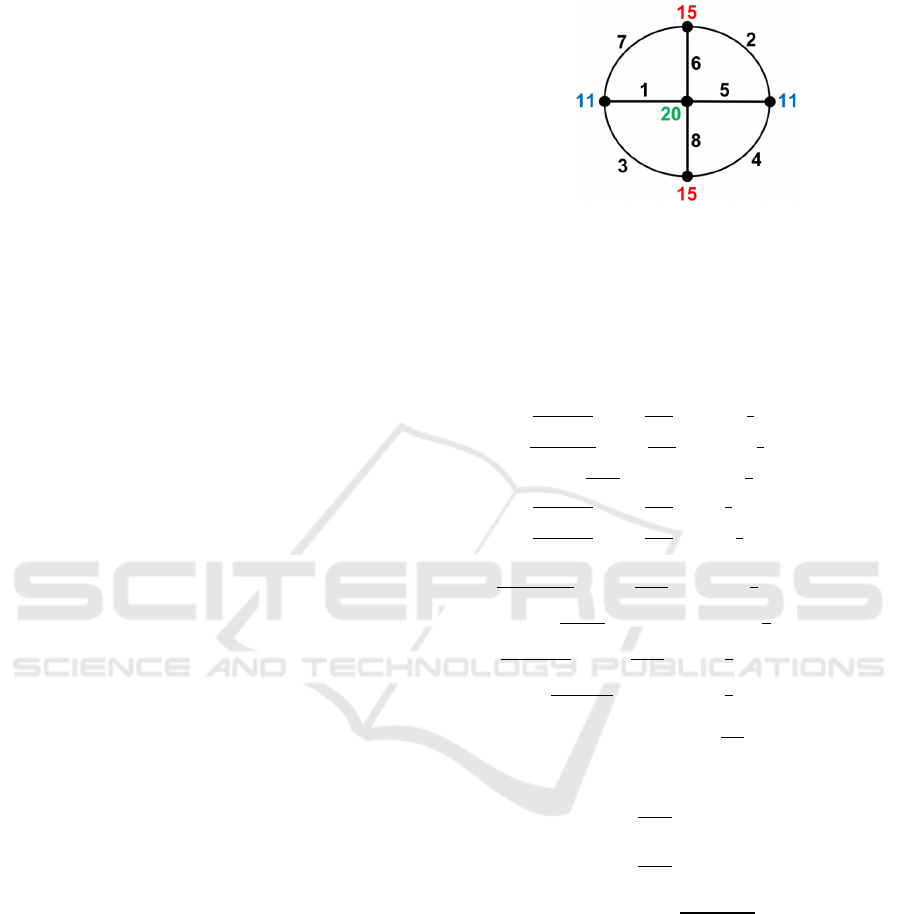
Case 1: 𝑛4

Figure 1: Local Antimagic Vertex Coloring of W4.

Label the edges of 𝑊



isomorphic to the

following figure. Therefore, for a small case of 𝑛

4, 𝜒





𝑊





3.

Case 2: 𝑛≡0 mod 8

Label the edges 𝑊



as follows

𝑓



𝑐𝑣







⎩

⎪

⎨

⎪

⎧

𝑛2𝑖10

𝑚



𝑖,3



𝑛4

,if 𝑖∈1,

𝑛

1



3𝑛 2𝑖 12

𝑚



𝑖,0



𝑛4

,if 𝑖∈2,

𝑛





3𝑛 4

,if 𝑖

𝑛

2,

3𝑛  2𝑖 6

𝑚



𝑖,3



𝑛4

,if 𝑖∈

𝑛

3,𝑛1



5𝑛  2𝑖 8

𝑚



𝑖,2



𝑛4

,if 𝑖∈

𝑛

4,𝑛



𝑓



𝑣



𝑣







⎩

⎪

⎨

⎪

⎧

15𝑛  2𝑖  2

𝑚



𝑖,1



𝑛4

,if 𝑖∈1,

𝑛

3



5𝑛  2𝑖

,if 𝑖∈2,

𝑛





7𝑛  2𝑖  4

𝑚



𝑖,0



𝑛4

,if 𝑖∈

𝑛

2,𝑛2



4𝑛𝑖1

,if 𝑖∈

𝑛

5,𝑛1



𝑓



𝑣



𝑣







5𝑛

The weights of vertices are

𝑤



𝑣









27𝑛

 1, if 𝑖 is odd,

29𝑛

2, if 𝑖 is even.

𝑤



𝑐





𝑛



𝑛1



It is clear that these three weights are distinct.

Therefore, 𝜒





𝑊





3 for 𝑛≡0 mod 8. We

conclude that 𝜒





𝑊





3 for 𝑛≡0 mod 8.

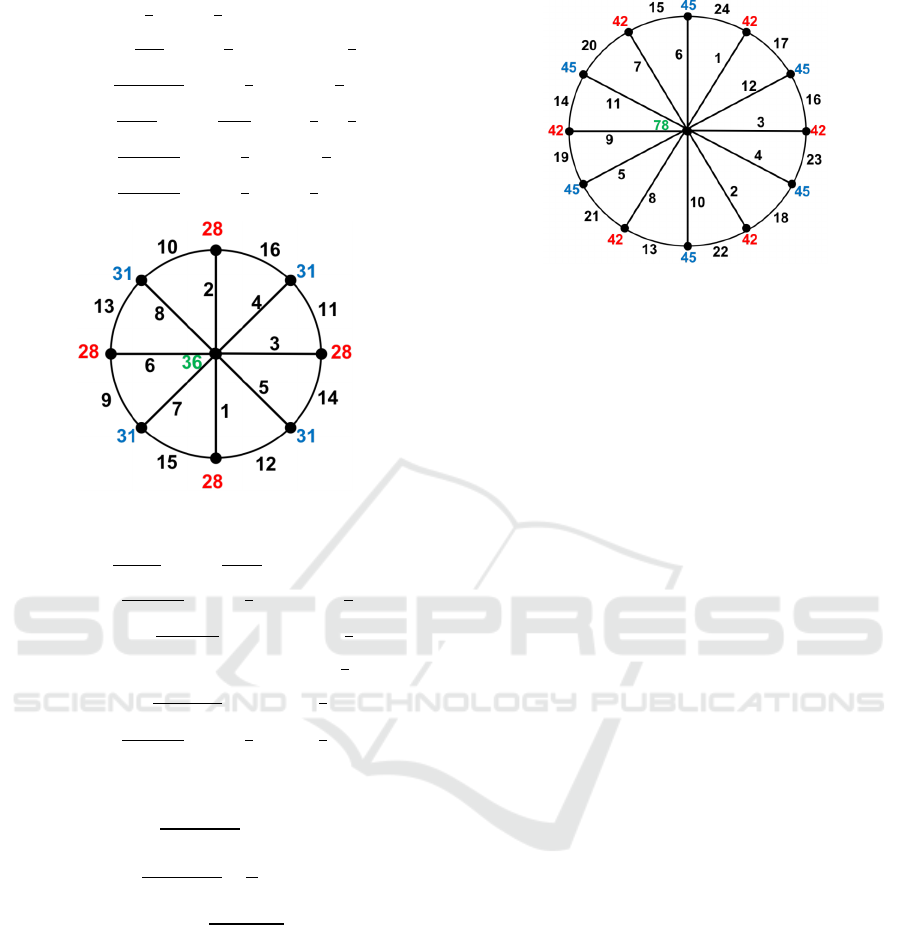
Case 3: 𝑛≡4 mod 8 and 𝑛12

Label the edges of 𝑊



as follows

IMC-SciMath 2019 - The International MIPAnet Conference on Science and Mathematics (IMC-SciMath)

186

𝑓



𝑐𝑣







⎩

⎪

⎨

⎪

⎧

𝑛

𝑚



𝑖,3



𝑛

,if 𝑖∈



1,3





𝑖2

𝑚



𝑖,0



𝑛

,if 𝑖∈2,

𝑛





2𝑛  2𝑖  2

𝑚



𝑖,3



𝑛

,if 𝑖∈5,

𝑛

1



3𝑛  4

𝑚



𝑖,1



𝑛2

,if 𝑖∈

𝑛

1,

𝑛

3



3𝑛𝑖4

𝑚



𝑖,2



𝑛

,if 𝑖∈

𝑛

2,𝑛



4𝑛𝑖1

𝑚



𝑖,3



𝑛

,if 𝑖∈

𝑛

5,𝑛1



Figure 2: Local Antimagic Vertex Coloring of 𝑊



𝑓



𝑣



𝑣







⎩

⎪

⎨

⎪

⎧

11𝑛  4

𝑚



𝑖,1



5𝑛  4

,if 𝑖∈



1,2



5𝑛𝑖1

𝑚



𝑖,1



𝑛

,if 𝑖∈3,

𝑛

1



4𝑛𝑖2

,if 𝑖∈4,

𝑛

2



𝑛  1, if 𝑖

𝑛

1,

5𝑛  2𝑖  2

,if 𝑖∈

𝑛

3,𝑛1



5𝑛𝑖6

𝑚



𝑖,0



𝑛

,if 𝑖∈

𝑛

4,𝑛2



The weights of vertices are

𝑤



𝑣









29𝑛 12

,if 𝑖 is odd,

29𝑛12



𝑛

,if 𝑖 is even.

𝑤



𝑐





𝑛



𝑛1



It is clear that these three weights are distinct.

Hence, 𝜒





𝑊





3 for 𝑛≡4 mod 8. We

conclude that 𝜒





𝑊





3 for 𝑛≡4 mod 8.

Figure 3: Local Antimagic Vertex Coloring of 𝑊



Therefore, f is a local antimagic vertex coloring for

𝑊



with 𝜒





𝑊





3.

∎

Helm graph is acquired by attaching a pendant to

every vertices in the wheel graph except the center.

Helm graph 𝐻



is formally defined with the vertex

set

𝑉



𝐻









𝑐,𝑣



,𝑥



|𝑖∈



1,𝑛



and the edge set

𝐸



𝐻









𝑣



𝑣



,𝑐𝑣



,𝑣



𝑣



,𝑐𝑣



,𝑥



𝑣



,𝑥



𝑣



|𝑖

∈



1,𝑛 1



We start to call vertex 𝑐 as a center, and vertices

𝑥



as pendants. In preceding theorem, we use the

chromatic number of the graph to prove the lower

bound. Next, we try to use reasoning similar with

Theorem 2 to establish the lower bound.

The center 𝑐 incident to 𝑛 number of edges. This

results the weight of center is at least the sum of

natural integers up to 𝑛. Meanwhile, the weight of

pendants is at most 3𝑛 since pendants incident to

only one edge. Vertices other than those are incident

to four edges, the weight is at least the sum of four

smallest available labels. By having assumptions and

showing contradictions, this reasoning effectively

adjusts the lower bound to be equal to the upper

bound, giving an exact value for helm graphs.

Theorem 4. For integer 𝑛3, helm graphs 𝐻



have

𝜒





𝐻







𝑛3, if 𝑛4,

6, if 𝑛4.

Proof. From the definition, helm graph 𝐻



has 𝑛

number of pendants. Using Theorem 2 directly, we

are guaranteed to have 𝜒





𝐻





𝑛1.

Let 𝑓 be a labeling of helm graph. We divide the

problems into cases.

Local Antimagic Vertex Coloring of Wheel Graph and Helm Graph

187

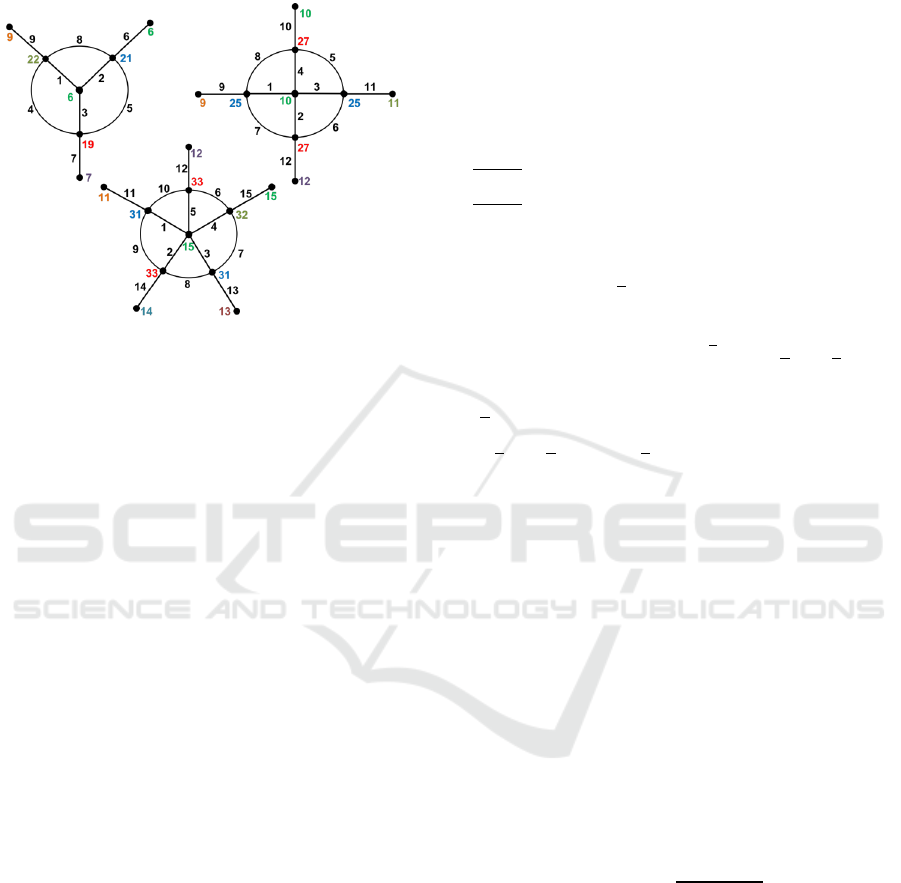
Case 1: 𝑛3,4,5

To prove the upper bound, labels the edges of 𝐻



isomorphic as the following figures.

Figure 4: Local Antimagic Vertex Coloring of 𝐻



, 𝐻



, and

𝐻



Hence, we have

𝜒





𝐻







6, if 𝑛3,4,

8, if 𝑛5.

Subcase 1.1: 𝑛3

Suppose 𝜒





𝐻





𝑛25. Then, there exists

𝑤



𝑣





that equals 𝑤𝑥



 for some 𝑖,𝑗. Notice that

every 𝑣



incident to four edges, which means

𝑤



𝑣





123410, if we chose smallest

labels on edges incident to 𝑣



. Meanwhile, 𝑤𝑥





9 because pendants only have one label. It

contradicts the fact the existence of 𝑤



𝑣





that

equals 𝑤𝑥



 for some 𝑖,𝑗. Therefore, 𝜒





𝐻







𝑛36. We conclude that 𝜒





𝐻





6.

Subcase 1.2: 𝑛4

Suppose 𝜒



𝑛1. Therefore, there exists two 𝑣



such that each one 𝑤



𝑣





equals to 𝑤𝑥



 for some

𝑖,𝑗. The sum of those 𝑤



𝑣







∑

𝑖





36. While

the sum of weights from pendants 𝑤



𝑥





2



3𝑛





6𝑛36. It contradicts the fact that each one 𝑤



𝑣





equals to 𝑤𝑥



 for some 𝑖,𝑗. Hence, 𝜒





𝐻





𝑛

26. We conclude that 𝜒





𝐻





6.

Subcase 1.3: 𝑛5.

A similar reasoning with previous case, that there is

no two 𝑣



such that each one 𝑤



𝑣





equals to 𝑤𝑥





for some 𝑖,𝑗 which means 𝜒



𝑛2. Suppose

𝜒



𝑛2. Therefore, there exists at least one 𝑣



and center 𝑐 such that each one 𝑤



𝑣





 𝑤𝑥



 and

𝑤



𝑐



𝑤



𝑥𝑘



for some 𝑖,𝑗,𝑘. Notice that 𝑤𝑥





15 for any 𝑗. There is only one possibility to satisfy

𝑤



𝑐



15, by giving 𝑐𝑣



labels from 1 to 5. Hence,

we have a new least weight of 𝑤



𝑣





156

719. It contradicts the fact that 𝑤



𝑣





𝑤𝑥





15 for some 𝑖,𝑗. Therefore, 𝜒





𝐻





𝑛3. We

conclude that 𝜒





𝐻





𝑛38.

Case 2: 𝑛6.

Suppose 𝜒





𝐻





𝑛1. Then, 𝑤



𝑐



will equal to

𝑤𝑥



 for some 𝑗. Notice that 𝑤



𝑐





∑

𝑖

















, while 𝑤𝑥



3𝑛 for any 𝑗. It is clear that











3𝑛, if 𝑛6. Therefore, contradiction

exists so that 𝜒





𝐻





𝑛2.

Suppose 𝜒





𝐻





𝑛2. Since 𝑤



𝑐



is unique,

then there exists 





 number of 𝑣



such that each one

𝑤



𝑣





equals to 𝑤𝑥



 for some 𝑖,𝑗. The sum of

those at least 𝑤



𝑣







∑

𝑖











2









4





1



While the sum of weights from pendants 𝑤



𝑥

















3𝑛



. It is not hard to prove the inequality

2









4





1













3𝑛



for 𝑛6, which

contradicts the fact that each one 𝑤



𝑣





equals to

𝑤𝑥



 for some 𝑖,𝑗. Hence, 𝜒





𝐻





𝑛3.

Subcase 2.1: 𝑛6, 𝑛 is even.

To prove the upper bound, labels the edges of 𝐻



follows

𝑓



𝑣



𝑣





𝑖, if 𝑖∈



1,𝑛 1



𝑓



𝑣



𝑣





𝑛,

𝑓



𝑐𝑣





2𝑛1𝑖, if 𝑖∈



1,𝑛



𝑓



𝑥



𝑣







2𝑛 2, if 𝑖1,

3𝑛𝑖12odd



𝑖



,if 𝑖∈



2,𝑛



The weights of the vertices are

𝑤



𝑥





𝑓𝑥



𝑣





𝑤



𝑣







5𝑛  1, if 𝑖 is even,

5𝑛  3, if 𝑖 is odd.

𝑤



𝑐





𝑛3𝑛 1

Therefore, 𝜒





𝐻





𝑛3 for 𝑛 is even. We

conclude 𝜒





𝐻





𝑛3 if 𝑛 is even.

IMC-SciMath 2019 - The International MIPAnet Conference on Science and Mathematics (IMC-SciMath)

188

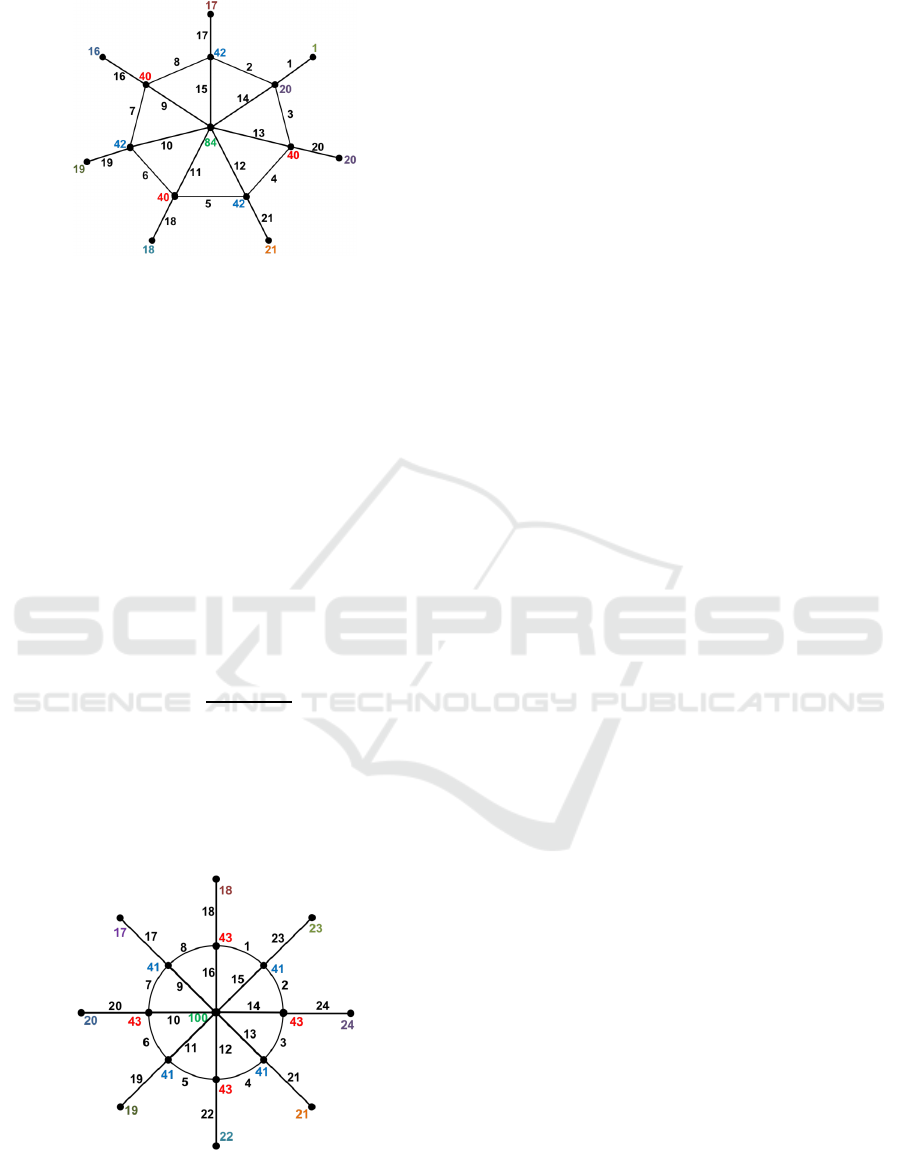
Figure 5: Local Antimagic Vertex Coloring of 𝐻



Subcase 2.2: 𝑛6, 𝑛 is odd.

To prove the upper bound, labels the edges of 𝐻



follows

𝑓



𝑣



𝑣





𝑖1, if 𝑖∈



1,𝑛 1



𝑓



𝑣



𝑣





𝑛1,

𝑓



𝑐𝑣





2𝑛2𝑖, if 𝑖∈



1,𝑛



𝑓



𝑥



𝑣







2𝑛  3, if 𝑖1,

1, if 𝑖2,

3𝑛𝑖22 odd



𝑖1



,if 𝑖∈



3,𝑛



The weights of the vertices are

𝑤



𝑥





𝑓𝑥



𝑣





𝑤



𝑣







2𝑛 6, if 𝑖2,

5𝑛  5, if 𝑖 is even and 𝑖2,

5𝑛 7, if 𝑖 is odd.

𝑤



𝑐





𝑛3𝑛 3

Notice that 𝑤



𝑣





𝑤



𝑥





. Hence, 𝜒





𝐻







𝑛3 for 𝑛 is odd. We conclude 𝜒





𝐻





𝑛3 if

𝑛 is odd.

Since every case is covered, then the theorem

holds.

∎

Figure 6: Local Antimagic Vertex Coloring of 𝐻



4 CONCLUSIONS

With preceding researchs, we have completed all

exact value of 𝜒





𝑊





and 𝜒





𝐻





for any integer

𝑛. Future researchers are recommended to study the

value of 𝜒



for any other class of graphs.

REFERENCES

Arumugam, S., Lee, Y., Premalatha, K., & Wang, T.

(2018). On Local Antimagic Vertex Coloring for

Corona Products of Graphs. ArXiv:1808.04956v1.

Arumugam, S., Premalatha, K., Baca, M., & Semanicová-

Fenovcíková, A. (2017). Local Antimagic Vertex

Coloring of a Graph. Graphs Combin., 33, 275–285.

Hartsfield, N., & Ringel, G. (1990). Pearls in Graph

Theory. Academic Press.

Haslegrave, J. (2018). Proof of a Local Antimagic

Conjecture. Discrete Mathematics and Theoretical

Computer Science, 20(1), 18.

Lau, G., Shiu, W., & Ng, H. (2018). On local antimagic

chromatic number of graphs with cut-vertices.

ArXiv:1805.04801v5.

Nazula, N. H., Slamin, S., & Dafik, D. (2018). Local

Antimagic Vertex Coloring of Unicyclic Graphs.

Indonesian Journal of Combinatorics, 2(1), 30–34.

Silaban, D. R., Pangestu, A., Herawati, B. N., Sugeng, K.,

& Slamin, A. (2009). Vertex-magic total labelings of

union of generalized Petersen graphs and union of

special circulant graphs. Combin. Math. and Combin.

Comput., 71, 201–207.

Local Antimagic Vertex Coloring of Wheel Graph and Helm Graph

189