A Study of Common Concerns Inhibiting Teacher Enactment of

Computational Thinking into Project-based Mathematics and Career

Technical Education

Subhrajit Majumder

1,*

, Kiyan Khaloozadeh

, Charlene M. Czerniak

, Jared Oluoch

Tod Shockey

, Ahmad Javaid

, Gale Mentzer

, Ryan Ducket

and Thehazhnan Ponnaiyan

Department of Electrical Engineering & Computer Science, University of Toledo,

2801 W Bancroft Street, Toledo, OH U.S.A.

College of Engineering, University of Toledo, 2801 W Bancroft Street, Toledo, OH, U.S.A.

Department of Engineering Technology, University of Toledo, 2801 W Bancroft Street, Toledo, OH, U.S.A.

Department of Teacher Education, University of Toledo, 2801 W Bancroft Street, Toledo, OH, U.S.A.

Acumen Research and Evaluation, 1811 N Reynolds Rd, Toledo OH, 43615, U.S.A.

Department of Chemical Engineering, University of Toledo, 2801 W Bancroft Street, Toledo, OH, USA

Keywords: MATH, STEM, PBL, CTE, CBAM.

Abstract: Recent studies have shown that US high school students are not as prolific as other countries in terms of their

performance in mathematics. One of the most effective solutions can be a change in the way mathematics

subjects is taught in high school. The NSF-funded “Understanding How Integrated Computational Thinking,

Engineering Design, and Mathematics Can Help Students Solve Scientific and Technical Problems in Career

Technical Education (INITIATE) project is a collaboration of The University of Toledo and high schools in

Toledo that aims to improve mathematics teaching. Project-based learning (PBL) and integrating math with

career technology education (CTE) have been established as efficient ways to improve high school students’

understanding of mathematics. Nevertheless, implementation of new ways of teaching is not always easy for

the teachers, and many factors may inhibit the teachers from implementing PBL methods. This research

analyzes common concerns teachers experienced regarding enacting new teaching methodologies in their

classroom. The Stages of Concern Questionnaire (SoCQ) was used to measure the teachers’ perceptions of

and comfort with implementing computational thinking (CT) concepts PBL lessons. Possible relationships

between teachers’ SoCQ CBAM score and other variables such as their understanding of PBL and CTE are

examined and discussed.

1 INTRODUCTION

The importance of mathematics preparation for

students pursuing higher education degrees is well

documented. Researchers at UCLA (2019) have

found that 60% of students entering community

colleges in the United States are not eligible for

college level mathematics courses. Based on

placement test results, these students arrive to

community colleges enrolling in remedial

mathematics courses. Enrolling in remedial

mathematics coursework increases time to degree,

which can lead to changes in degree pursuit

This project is funded by the National Science Foundation

Grant Number 1741784.

(WOLPERT, 2018). The Bureau of Labor Statistics

(Employment in STEM occupations : U.S. Bureau of

Labor Statistics, n.d.) reports that Science,

Technology, Engineering, and Mathematics (STEM)

employment rates are low when compared to overall

employment in the United States. One finding that

may contribute to a lack of engagement in

mathematics by students in secondary school may be

mathematics anxiety.

According to Maloney and Beilock, mathematics

anxiety may also be a product of poor mathematics

skills due to a lack of mathematical practice, which

may lead to a lack of conceptual understanding

(Beilock & Maloney, 2015). Mathematics anxiety is

Majumder, S., Khaloozadeh, K., Czerniak, C., Oluoch, J., Shockey, T., Javaid, A., Mentzer, G., Ducket, R. and Ponnaiyan, T.

A Study of Common Concerns Inhibiting Teacher Enactment of Computational Thinking into Project-based Mathematics and Career Technical Education.

DOI: 10.5220/0009337403410349

In Proceedings of the 12th International Conference on Computer Supported Education (CSEDU 2020) - Volume 1, pages 341-349

ISBN: 978-989-758-417-6

341

being experienced by many learners. Anxiety for too

many students is expressed as “that feeling of fear,

apprehension, and helplessness when tackling a math

problem” (TRAN, n.d.). Tran citing the work of

Brooks (2014) discusses the possibility of turning

“anxiety into excitement”(Brooks, 2013). Brooks

(2014) researched how anxiety and excitement are

linked to increased heart rates but have different

psychological effects. Excitement, according to

Brooks is related to “I can do something” while

anxiety can lead to a “threat mindset.” Prior to

presenting a difficult mathematics problem,

participants in Brooks’ study were shown the

message “try to get excited.” This message resulted in

improved performance over the messages of “try to

remain calm” or “please wait a few minutes.”

The UCLA group (2019) reported that one

difficulty for students is a lack of a deep

understanding of the mathematics they study. This

lack of understanding may be attributed to the

memorization of mathematical rules and procedures

according to the group(WOLPERT, 2018). Paulos

(1991) put this in perspective:

Imagine that 90 percent of every

course in English up until college was

devoted to grammar and the diagramming

of sentences. Would graduates have any

feeling for literature? Or let’s consider a

conservatory devoting around 90 percent

of its effort to only practicing of the

scales. Would this way be good enough

for the students to develop understanding

or appropriate appreciation of music?

Obviously, the answer is no. In fact, this

gives proper allowances for the

hyperbole. This describes what frequently

occurs in our mathematics classes.

Mathematics is identified with a rote

recitation of facts and a blind carrying out

of procedures. (p. 52)(Paulos, n.d.).

Schools across the world are beginning to address this

lack of deep conceptual understanding according to

(P. Lisette et al., 2012). Addressing conceptual

understanding supports students to solve new

mathematical problems and to make connections

within mathematical concepts (Macmath et al., 2009).

This paper addresses a professional development

program that partners secondary school mathematics

teachers with university engineers, science educators,

and mathematics educators. Through this partnership

we are striving toward exciting mathematics

classrooms where teachers engage students in new

mathematical problems, the exploration of

connections within mathematics and mathematics to

other subject areas, through the study of autonomous

vehicles.

2 ISSUES WITH HOW

TEACHERS TEACH

Many teachers across the globe are going through

professional development with the goal of better way

of teaching and these are being proved successful. For

example, most teachers with this expertise cover

around 40 problems in a day through various types of

games, drills, or written work whereas amateur

teachers cover around 6-7 problems only (Wilson et

al., 2005).

2.1 PBL as a Promising Approach

According to several research studies, problem-based

learning (PBL) is considered to be a compelling

possibility to enhance students’ ability to perceive

and solve mathematical problems (Tarmizi et al.,

2010). Through PBL, students learn to develop their

critical thinking and, as a result, create a foundation

for the application of skills to new situations. Authors

in (Han et al., 2015) have investigated whether

participating in STEM PBL activities effected

students who had varied performance levels and to

what extent students’ individual factors influenced

their mathematics achievement. Since STEM PBL

embodiment in schools has been a critical challenge,

the effect of STEM PBL on various factors should be

examined. Teachers from 3 highs schools participated

in sustained professional development training

conducted by a STEM centre based in a Southwestern

University. They were asked to develop STEM based

PBL lesson plans once in every 6 weeks for a period

of 3 years(Han et al., 2015). 836 high school students

participated in Texas Assessment of Knowledge and

Skills (TAKS) test at least in the initial year. The

scores were analysed along with demographic

information by hierarchical linear modelling to

project the longitudinal study. The results show that

student achievements in mathematics by both

demographic backgrounds and performance levels

were influenced by STEM PBL instruction. Over the

3 years of this experiment, the students with low

performing skills showed significantly better

improvement than high and middle performing

students.

Another promising way is integration of Math in

CTE. Despite the fact the combination is not a

CSEDU 2020 - 12th International Conference on Computer Supported Education

342

curriculum, this strategy has been proved successful

at several high schools as this led to increased

academic engagement and achievement for students

(Newmann Editor, n.d.). Invoking PBL, putting Math

and CTE together and other established promising

techniques could have eradicated the whole issue of

“high school students lagging in Mathematics” by

their own. Except there is a major concern which

causes several other significant obstacles for this

prosperous journey.

2.2 Issues Related to Making Change

in Classrooms

As interaction with students in a classroom is the

major factor for them to learn, changes in the way of

teaching is one of the major steps. Students must find

fun in their studies to improve their ability to solve

mathematical problems and it is teachers’

responsibility to make learning interesting and fun for

the students. Although there might be no teacher who

will not value collaboration, creativity and curiosity

in their classrooms, many classes are devoid of these

very traits (Herrmann, 2017). From the past

experiments it is given that the results of

experimenting changes in high school curriculums

are equivocal.

Aguirre and Speer adopted an inclusive view of

beliefs as “conceptions, personal ideologies, world

views and values that shape practice and orient

knowledge”(Aguirre & Speer, 1999). Two important

aspects of beliefs get highlighted by this view which

receive general agreement among researchers and are

relevant to the current study. First is the conviction

that beliefs and behaviors are inherently linked (Di

Martino & Zan, 2011), (Forgasz & Leder, 2008).

While Ernest (Ernest, 1989) and Furinghetti and

Morselli in (Furinghetti & Morselli, 2011) consider

beliefs to be the main regulators of teachers’

practices, others acknowledge the general influence

they have on teachers’ pedagogical decision-making

(Goldin, 2009). Second is the relationship between

knowledge and beliefs. As teachers’ beliefs play a

significant role in conducting their practices suggests

that their beliefs act as subjective knowledge;

“knowledge” that the teachers believe to be true but

actually it is not (Beswick, 2011). Their beliefs play

one of the most significant roles in their classrooms.

Hence, teachers’ beliefs are considered to be the

fundamental factor to the investigations of teaching

and learning mathematics. While many

categorizations of beliefs exist in the literature, they

can be broadly grouped according to beliefs about the

discipline, its teaching and student learning (Cross,

2009). Often researchers who study teachers’ beliefs

focus on a cluster of related beliefs, such as their

beliefs about teaching proof (Furinghetti & Morselli,

2011). In (Bobis et al., 2016) teachers’ mathematical

beliefs about student engagement relating to the

discipline of mathematics were examined,

mathematics teaching and learning, and about

themselves as teachers and learners of mathematics.

As far as we are aware, there are few studies that

focus on the priority the teacher places on the

intervention in relation to his/her job as a teacher.

2.3 Common Reasons Change Does Not

Happen in Classrooms

“Taking a new step, uttering a new world, is what

people fear most”-Fyodor Dostoevsky. People get

accustomed to things over time, similarly teachers are

also accustomed to orthodox curriculum standards

and due to normal human behaviour adapting a

change in those might cause hiccups. Some states

claim the possibility of Common Core State

Standards (About the Standards | Common Core State

Standards Initiative, n.d.) eliminate the need for

students to undergo remedial courses upon admission

to postsecondary institutions within the system. This

claim can stand as an excuse to bypass these

standards. However, there are some states which try

to update their curriculum standards to help students

improve their skills for their career. In this the authors

have experimented work-based learning experience

to improve English and Mathskills of physically

challenged students (Cease-Cook et al., 2015).

2.4 Concerns Teachers Have

At the time of implementing those changes in

classrooms, a major issue comes into play: teachers’

beliefs (Handal & Herrington, 2003). They rely on

their beliefs more than on going trend in pedagogy.

Herrman, (Herrmann, 2017) presented possible

resistances to changes for teachers: A traditional

sense of one’s own competence, the comfort of

predictability, and familiar successes. When a teacher

is asked to apply changes in their classroom, it also

changes the way they see themselves. They face fear

to go out of their comfort zones where they lack

confidence. Also, when teachers make some

innovative moves, success is not guaranteed. They

will face failure inevitably as not every experiment

will be successful. Last but not the least, the author

also pointed out how asking teachers to leave their

comfortable lesson plans behind for a new

environment in which the students may struggle may

A Study of Common Concerns Inhibiting Teacher Enactment of Computational Thinking into Project-based Mathematics and Career

Technical Education

343

create hindrance. The change in attitudes of 29 self-

selected middle and high school teachers towards

interdisciplinary teaching is described in (Al Salami

et al., 2017). The teachers went through a profession

Development (PD) and delivered interdisciplinary

teaching for 12-15 week. Over these weeks they

designed problem units which spanned multiple

STEM subjects. Quasi-experimental pilot study had

been made by the researchers. This study used several

survey methods and implemented a single group pre-

test and post-test design from the data collected at two

intervals; first one was done at the time of PD

workshop and the later one was conducted after the

completion of the teaching unit which emphasized a

long-term engineering design problem. The goals of

this research were:

 Assess the changes in attitudes to

interdisciplinary teaching, attitudes to

teamwork, teaching satisfaction, and

resistance to change.

 Explore relationships among these

changes.

 Describe the variation in these changes

across teachers’ gender, school level,

discipline taught, and education level.

2.5 Concerns-Based Adoption Model

(CBAM) as an Approach for

Identifying and Remedying

Concerns

Human relation in curriculum change has proved its

value for individuals and groups interested in the

improvement of education (Benne & Muntyan, n.d.).

Charalambous et.al (Charalambous & Philippou,

2010) have analysed data collected from 151

elementary mathematics teachers. They examined

how teachers’ beliefs and efficacy beliefs come into

play when mandatory changes occur in traditional

mathematic curriculums. Some researchers have

utilized anecdote circles, storytelling via moderated

group discussions, to investigate teachers’ needs

related to developing and implementing authentic,

interdisciplinary PBL activities in an urban, public

STEM high school (deChambeau & Ramlo, 2017).

The experiences and viewpoints of teachers towards

this approach were explored within three broad

themes: assessment; coaching and training; and

authentic learning. These analysis delivers insights

for implementing PBL, improving teaching and

learning best practices in a school.

The integration of STEM subjects offers students

opportunities to solve real-world problems in real-

world-like situations (Tsupros et al., n.d.) where

knowledge is used as a tool to solve problems rather

than a body of facts or procedures to be learned with

little contextual significance (Herschbach, 2011).

Despite the growing emphasis on and demonstrated

importance of integration in STEM education,

teachers and teacher educators are not typically

trained to work in areas that rely on the integration of

multiple disciplines. Thus, teachers have not likely

experienced integration themselves and are not well

prepared to engage students in the cross-disciplinary

learning called for by the latest national standards

documents in math and science, such as the Next

Generation Science Standards (Krajcik et al., 2014)

and Common Core State Standards for Mathematics

(Branding Guidelines | Common Core State

Standards Initiative, n.d.). STEM teachers may face

several challenges when they attempt to integrate

content from different disciplines. These challenges

include (a) knowledge of disciplinary specific

differences between subject areas (Lederman &

Lederman, 2014), (b) a lack of breadth in their own

content knowledge needed for teaching (Loewenberg

Ball et al., 2008) in multiple subject areas, and (c) the

contextual challenges of co-planning and/or co-

teaching across disciplinary boundaries (Berlin &

White, 2010), (Frykholm & Glasson, 2005).

This project (INITIATE) combines multiple

theories (the fusion of activity theory, social

constructivist learning theory, and project-based

learning) to form its conceptual framework or

approach to address this concern. For the guidance of

professional development using problem-based

learning to make grade 9-12 science teachers capable

to integrate Computational Thinking into their

teaching, this project uses the conceptual framework

of Concerns-Based Adoption Model (CBAM).

Activities will use smart vehicles as a mechanism to

engage mathematics teachers in Career Technical

Education, alongside with 9-12 students to better

understand why and how to embed computational

thinking in their curriculum. The program should

contribute meaningfully to the understanding of

effective characteristics of professional development.

Funded by the STEM Computing program, this

project seeks to address emerging challenges in

computational STEM areas. The project integrated

computational thinking with computing activities

within disciplinary STEM teaching and learning in

early childhood education through high school (preK-

12).

CSEDU 2020 - 12th International Conference on Computer Supported Education

344

3 METHODOLOGY

We have used several instruments and tools such as

Teacher Lesson Plans, Teacher concerns with

enactment of CT, Focus Group Interviews, etc to

create the CBAM model for the participating

teachers.

When an innovative idea is introduced to a group

of people, the initiative demands not only the

provision of materials, resources, and training; the

understanding of how each person will react to the

new initiative with different attitudes and beliefs is

also vital. “The instrument which is used to evaluate

the efficacy of the Understanding by Design

instructional framework for the implementation plan

is called Concerns Based Adoption Model (CBAM)”

(Hall Richard C Wallace & William Dossett, 1973).

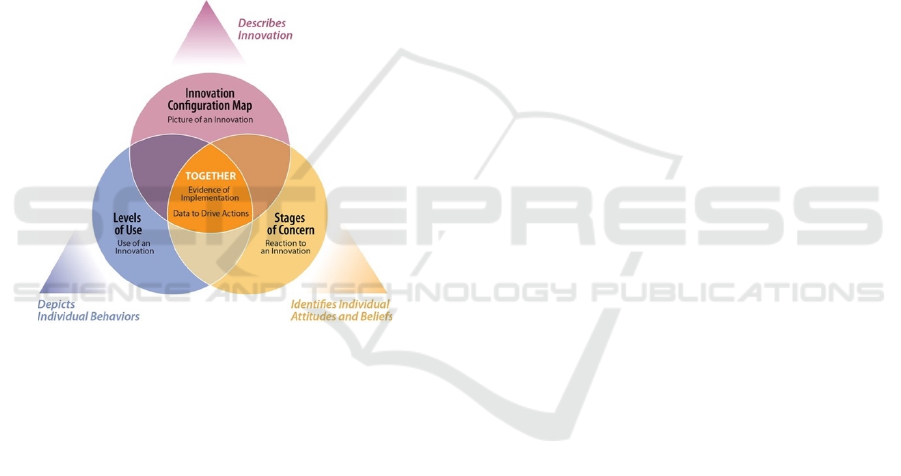
Figure 1: CBAM Model (Concerns-Based Adoption Model

(CBAM), n.d.).

This model provides techniques and tools for

accessing and facilitating the implementation of new

ideas, innovations and reform initiatives (Concerns-

Based Adoption Model (CBAM), n.d.). CBAM can be

divided into three diagnostic dimensions as follows:

 Innovation Configuration:

Innovation Configuration allows the teacher to

provide feedback on the implementation of

different types of innovation in the classroom.

The teachers could as well realize what

adjustments could be made to their teaching

behaviour to decrease the difficulty level of the

content (Hall & Hord, n.d.). This allows

evaluators to monitor the results obtained from

the teachers to use an innovation appropriately

in the future.

 Stages of Concern:

The Stages of Concerns (SoC) process, which

includes a questionnaire, interview, and open-

ended statements, enables leaders to identify

staff members’ attitudes and beliefs toward a

new program or initiative. With this

knowledge, leaders can take actions to address

individuals’ specific concerns. The SoC items

discussed in this work are as follows:

o Unconcerned: “I have heard about this

but don’t have time to put effort in it.”

o Informational: “This looks promising,

maybe I would like to read about it to

know better.”

o Personal: “The changes I have to make

in my daily schedule is making me

concerned.”

o Management: I am concerned about how

much effort it is going to consume to

become a hit.”

o Consequence: “If I successfully make

this project run, how it is going to affect

my students.”

o Collaboration: “I would like to share

these ideas with others also.”

o Refocusing: “Maybe this approach will

give better results than the proposed

one.”

 Levels of Use:

Levels of Use (LoU) analyzes teacher

behaviors from the start of making changes in

their classroom. It indicates the magnitude and

amount of change as the teachers go through

with their teaching transition (Horsley &

Susan, 1998). Each level of the transition is

identifiable by a key decision point and its own

behavioural characteristics (Powell-Griner et

al., 1997).

In this work, we have looked into the stages of

concern encountered by the teachers from this

INITIATE project. As teachers hold the prime

deciding factor for any changes tried to make in

instructional planning and content, their behaviour

analysis must be the first step. How they react to this

change in their classroom, are they comfortable with

this new way of teaching or do the accustomed ways

seem more worthwhile to them-these are among those

vitals questions which have to be answered before

moving forward.

A Study of Common Concerns Inhibiting Teacher Enactment of Computational Thinking into Project-based Mathematics and Career

Technical Education

345

4 RESULTS

Sixteen participant teachers completed the first and

second administration of the SoCQ (one teacher had

difficulty accessing the survey and did not complete

it). As a reminder, SoCQ is divided into three major

constructs: concern about impact, concern about the

task of implementing (logistics), and concern about

self (self-efficacy). Respondents are given a series of

statements and are asked, using a 7-point scale to

indicate their level of agreement with the statement.

Anchors within the scale are:

 7 = true most of the time

 4 = true some of the time

 1 = not true at all at this time

 0 = this statement is not relevant to me

A score of 0 indicates that the innovation is not a high

priority to the respondent. There are six stages of

concern and they are illustrated in Figure 2.

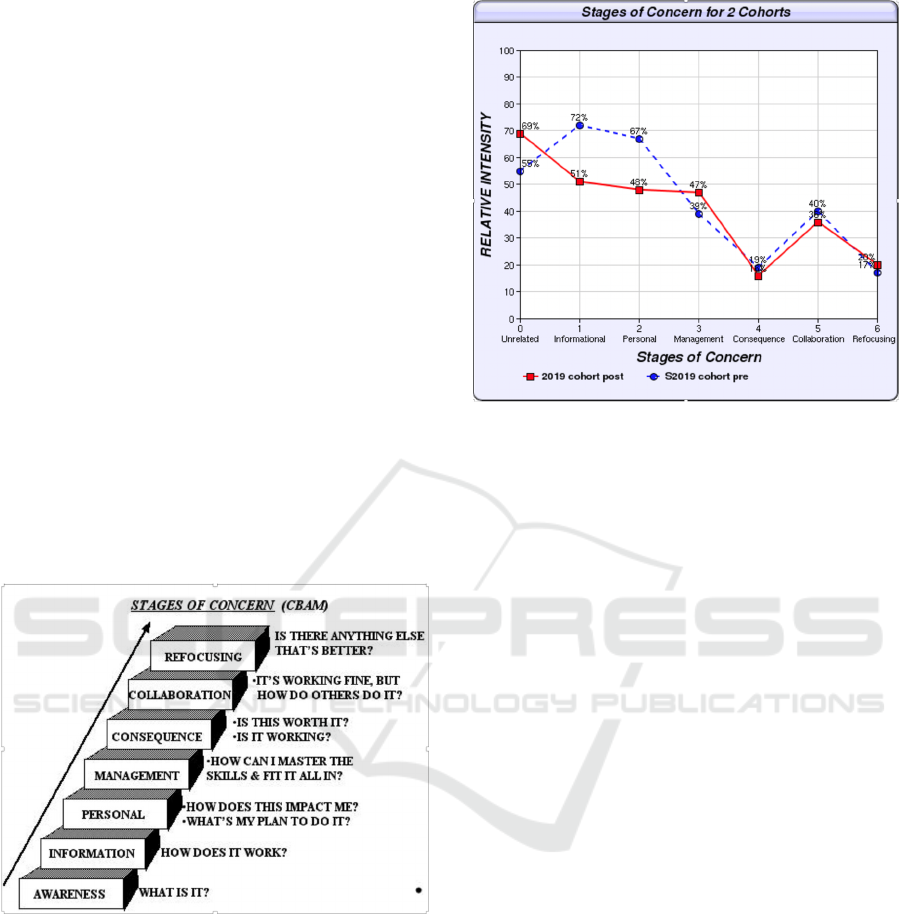
Figure 2: Stages of Concern Scales.

The stages are developmental in that one progress

from the lowest “step” to the highest as he/she

becomes more comfortable implementing the

innovation.

Figure 2 illustrates the group distribution of the

2019-20 cohort. The first administration was

completed on the first day of the Summer Institute

and the second administration was in January 2020—

approximately 6 months after completing the Institute

and after (in most cases) implementing a lesson that

based upon Summer Institute content.

Figure 3: Group Distribution of the cohort 2019-2020.

Notice that the post scores show the group moving

lower on the Informational and Personal scales

(interest in the INITIATE model but not quite sure it

was relevant to their teaching and need for more

information regarding the specifics of the innovation

before being willing to implement, respectively) and

slightly higher on the unrelated category suggesting

that they have gained some information needed to

implement the lessons but in some cases this

information has moved them to a position where they

feel the INITIATE teaching strategies are not relevant

to what they do in the classroom. The remaining four

categories have remained relatively similar on pre and

post testing. The low score for Consequences

indicates that the teachers as a group do not have

concerns as to how the innovation might affect the

students, particularly adversely. Similarly, the low

score on the Refocusing scale suggests that the

teachers have little interest in refining and adjusting

the INITIATE teaching approach to better serve

students and make it more useable by other teachers.

Overall, the main conclusion that can be drawn is that

the teachers’ concerns about implementing

INITIATE teaching strategies has not changed much

over the past six months.

Individual change can provide insight as to how

teacher concerns might be addressed to help them

better embrace the innovation. Table 1 (next page)

illustrates the individual percentile scores for the

Stages on pre and posttest. Cells highlighted in

yellow indicate the highest percentile for each testing

occasion per individual. As recommended by the

Stages of Concern Instrument Manual, when another

stage score is within one or two percentile points of

CSEDU 2020 - 12th International Conference on Computer Supported Education

346

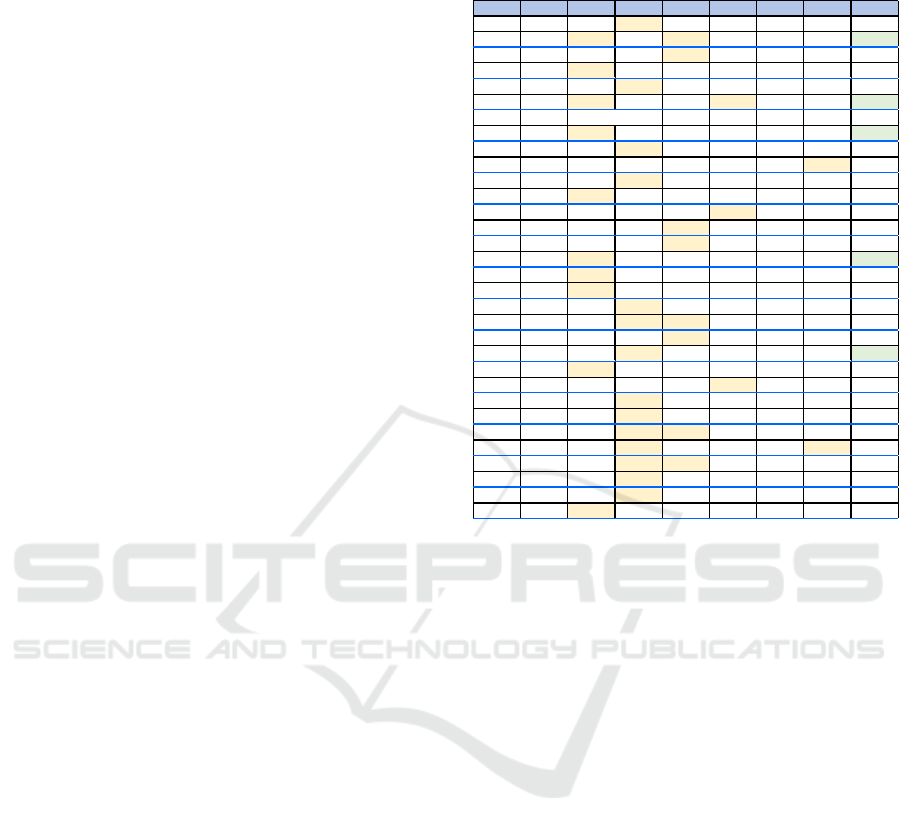
the highest score, both scores have been highlighted.

Concerning the adoption of an innovation, the typical

non-user profile will have high scores for Stages 0 –

2 and low scores for 4 -6. The typical user will have

the highest score at Stage 3 or above. Stage numbers

represent the following stages:

0 = unconcerne

1 = Informational

2 = Personal 3 = Mana

ement

4 = Consequence 5 = Collaboration

6 = Refocusin

As expected, nearly all the teachers scored as

nonusers on the pretest (one scored in the 3

category). Of the cohort, five were CTE teachers, one

was a special education teacher, and the remaining

were math teachers. Two teachers (24 and 28) scored

in the Unconcerned category indicating they felt the

innovation was not a priority. The one (ID 20) who

scored as a user, was a math teacher. On the posttest,

3 remained nonusers, one (20) slipped from user to

nonuser (most likely due to preconceptions prior to

the Institute), two were split between user and

nonuser status (16 and 30), and two progressed to

users (18 and 28). Eight teachers scored in the

Unconcerned category. This category does not

indicate whether the teacher is actually a user of the

innovation but rather indicates that the innovation is

low in priority when compared to other tasks

associated with teaching. Of the eight, seven moved

from a higher category on the pretest to Unconcerned

on the posttest. One (16) was split between

Management (concern with time and facility

management and how the teaching strategies might fit

into the class period) and Unconcerned. This suggests

that 16 wants to implement INITIATE strategies but

is concerned about managing it especially

considering other teaching responsibilities.

Respondent 30 was split between Refocusing and

Information suggesting that this teacher has ideas of

ways to modify the innovation but still needs more

information about how it works.

higher score for Stage 6 than for Stages 4 and/or

5 indicates that the respondent has ideas that have

more merit than the proposed innovation. Scores

highlighted on the posttest in Stage 6 in light green

are such occasions. Five teachers fell into this

category.

Table 1: individual percentile scores for the Stages on pre

and posttest.

Looking at the individual scores as a whole, it appears that

many of the teachers feel INITIATE teaching strategies are

not a priority and, most likely, they are targeting the

implementation of the lessons they developed using the

self-driving model cars. In addition, several still feel the

need for more information regarding how to implement the

strategies/lessons. Others, those who ranked at the Personal

level, may have doubts as to whether they are able to

implement the lessons correctly.

Delving more into what kinds of information the

teachers need as well as their reservations about

implementing the lessons could provide insight as to the

direction of future teacher support sessions. There has been

some difficulty with the technical aspects of using the cars

and that may be contributing to the doubt some teachers

have. Exploring that as well as other types of support that

could be useful may alleviate teacher concerns.

5 FUTURE WORK AND

CONCLUSION

In this study, the behavioral changes of high school

teachers integrating PBL and Curriculum Technical

Education (CTE) in their lesson plans are analyzed

and assessed using CBAM scoring system.

INITIATE is a National Science Foundation (NSF)

program about Autonomous Vehicles that utilizes

CTE and PBL in its lesson plans, integrating these

concepts in high schools teaching curriculums. The

ID 0123456

10Pre 48756323 919 9

Post 48 45 48 34 13 22 26

11Pre 146667473828 9

Post 99 51 57 65 44 28 38

16Pre 61665923214017

Post 94 34 25 94 3 5 20

17 Pre No pretest data

Post 75 34 28 47 5 9 14

18Pre 149076432452 9

Post 14 60 45 23 8 68 14

19Pre 145139271322 9

Post 31 27 28 15 9 9 9

20Pre 81697685244460

Post 22 93 80 30 59 25 11

21Pre 55697869637252

Post 91 60 59 65 38 31 52

24Pre 99666730116417

Post 87 60 59 60 11 68 17

25Pre 31787634165547

Post 81 96 97 30 21 93 52

27Pre 487578271359 3

Post 40 57 21 30 8 22 26

28Pre 99604834 3 7 9

Post 91 37 55 95 54 55 30

29Pre 40696730213620

Post 14 43 41 15 8 22 9

30 Pre 7 54 55 39 13 25 9

Post 55 60 35 34 5 59 2

31 Pre 7 75 76 11 7 25 9

Post 14 27 25 15 11 16 9

32Pre 40976369387247

Post 75 45 45 30 21 52 38

A Study of Common Concerns Inhibiting Teacher Enactment of Computational Thinking into Project-based Mathematics and Career

Technical Education

347

Stages of Concern Questionnaire (SoCQ) was used to

measure the teachers’ perceptions of and comfort

with implementing computational thinking (CT)

concepts PBL lessons. Based on the observations

gained from the teacher implementation of the lesson

plans, the pre cohort and post cohort results follow the

expected behavioral line in the given graphs.

Furthermore, the use of technology and integrating it

into the lesson plans does indeed bring benefits, but it

also causes problems of its own that hinder the use of

PBL and CTE concepts in high schools. For instance,

if halts occur to the technology, it will require special

assistance to fix the errors and it also can cause large

delays while teaching the lesson. The initial lessons

implementing PBL and CTE topics can be monitored

to make sure that they are on the right track. Also, a

designated tech-savvy person could always be placed

in the classroom for assistance with any problem.

REFERENCES

About the Standards | Common Core State Standards

Initiative. (n.d.). Retrieved October 26, 2019, from

http://www.corestandards.org/about-the-standards/

Aguirre, J., & Speer, N. M. (1999). Examining the

relationship between beliefs and goals in teacher

practice. Journal of Mathematical Behavior, 18(3),

327–356. https://doi.org/10.1016/s0732-

3123(99)00034-6

Al Salami, M. K., Makela, C. J., & de Miranda, M. A.

(2017). Assessing changes in teachers’ attitudes toward

interdisciplinary STEM teaching. International Journal

of Technology and Design Education, 27(1), 63–88.

https://doi.org/10.1007/s10798-015-9341-0

Beilock, S. L., & Maloney, E. A. (2015). Math Anxiety.

Policy Insights from the Behavioral and Brain

Sciences, 2(1), 4–12. https://doi.org/10.1177/

2372732215601438

Benne, K. D., & Muntyan, B. (n.d.). Human relations in

curriculum change dryden press professional books in

education human relations in curriculum change.

Berlin, D. F., & White, A. L. (2010). Preservice

mathematics and science teachers in an integrated

teacher preparation program for grades 7-12: A 3-year

study of attitudes and perceptions related to integration.

International Journal of Science and Mathematics

Education, 8(1), 97–115. https://doi.org/10.1007/

s10763-009-9164-0

Beswick, K. (2011). Knowledge/Beliefs and their

relationship to emotion.

Bobis, J., Way, J., Anderson, J., & Martin, A. J. (2016).

Challenging teacher beliefs about student engagement

in mathematics. Journal of Mathematics Teacher

Education, 19(1), 33–55. https://doi.org/10.1007/

s10857-015-9300-4

Branding Guidelines | Common Core State Standards

Initiative. (n.d.). Retrieved February 27, 2020, from

http://www.corestandards.org/about-the-

standards/branding-guidelines/

Brooks, A. W. (2013). Get Excited: Reappraising Pre-

Performance Anxiety as Excitement. https://doi.org/

10.1037/a0035325

Cease-Cook, J., Fowler, C., & Test, D. W. (2015).

Strategies for Creating Work-Based Learning

Experiences in Schools for Secondary Students With

Disabilities. TEACHING Exceptional Children, 47(6),

352–358. https://doi.org/10.1177/0040059915580033

Charalambous, C. Y., & Philippou, G. N. (2010). Teachers’

concerns and efficacy beliefs about implementing a

mathematics curriculum reform: Integrating two lines

of inquiry. Educational Studies in Mathematics, 75(1),

1–21. https://doi.org/10.1007/s10649-010-9238-5

Concerns-Based Adoption Model (CBAM). (n.d.).

Retrieved February 27, 2020, from http://

www.sedl.org/cbam/

Cross, S. (2009). Adult Teaching and Learning: Developing

Your Practice - Sue Cross - Google Books.

https://books.google.com/books?hl=en&lr=&id=PAB

FBgAAQBAJ&oi=fnd&pg=PP1&dq=Cross+2009+tea

ching+and+student+learning&ots=hhKnIi-PQ0&sig=-

2_piMlr7_3Kf4iNNW1rKBkX5ow#v=onepage&q=Cr

oss 2009 teaching and student learning&f=false

deChambeau, A. L., & Ramlo, S. E. (2017). Stem high

school teachers’ views of implementing PBL: An

investigation using anecdote circles. Interdisciplinary

Journal of Problem-Based Learning, 11(1). https://

doi.org/10.7771/1541-5015.1566

Desilver, D. (n.d.). U.S. academic achievement lags that of

many other countries | Pew Research Center. Retrieved

February 26, 2020, from https://www.pewresearch.org/

fact-tank/2017/02/15/u-s-students-internationally-

math-science/

Di Martino, P., & Zan, R. (2011). Attitude towards

mathematics: A bridge between beliefs and emotions.

ZDM - International Journal on Mathematics

Education, 43(4), 471–482. https://doi.org/10.1007/

s11858-011-0309-6

Employment in STEM occupations : U.S. Bureau of Labor

Statistics. (n.d.). Retrieved February 26, 2020, from

https://www.bls.gov/emp/tables/stem-employment.htm

Ernest, P. (1989). The Knowledge, Beliefs and Attitudes of

the Mathematics Teacher: a model. Journal of

Education for Teaching, 15(1), 13–33. https://doi.org/

10.1080/0260747890150102

Forgasz, H., & Leder, G. (2008). Beliefs about mathematics

and mathematics teaching | Request PDF.

https://www.researchgate.net/publication/288945467_

Beliefs_about_mathematics_and_mathematics_teachin

Frykholm, J., & Glasson, G. (2005). Connecting Science

and Mathematics Instruction: Pedagogical Context

Knowledge for Teachers. School Science and

Mathematics, 105(3), 127–141. https://doi.org/

10.1111/j.1949-8594.2005.tb18047.x

CSEDU 2020 - 12th International Conference on Computer Supported Education

348

Furinghetti, F., & Morselli, F. (2011). Beliefs and beyond:

Hows and whys in the teaching of proof. ZDM -

International Journal on Mathematics Education,

43(4), 587–599. https://doi.org/10.1007/s11858-011-

0316-7

Goldin, C. D. (2009). The Race between Education and

Technology - Claudia Dale Goldin, Lawrence F. Katz -

Google Books. https://books.google.com/

books?hl=en&lr=&id=yGlCFqnakCoC&oi=fnd&pg=P

R5&dq=Goldin+et+al.+2009&ots=HtGnUpOOMV&s

ig=4UxD69rIdbEJGLFm1384YrY93RE#v=onepage&

q=Goldin et al. 2009&f=false

Hall, G. E., & Hord, S. M. (n.d.). Implementing Change:

Patterns, Principles, and Potholes. Retrieved February

27, 2020, from www.mathedleadership.org

Hall Richard C Wallace, G. E., & William Dossett, J. F.

(1973). A developmental conceptualization of the

adoption process within educational institutions this

document has been repro ouced exactly as received

from the person or organization origin ating it points of

view or opinions stated do not necessarily repre sent

official national institute of education position or policy

ill) research and development center for teacher

education.

Han, S., Capraro, R., & Capraro, M. M. (2015). How

science, technology, engineering, and mathematics

(stem) project-based learning (pbl) affects high, middle,

and low achievers differently: the impact of student

factors on achievement. International Journal of

Science and Mathematics Education, 13(5), 1089–

1113. https://doi.org/10.1007/s10763-014-9526-0

Handal, B., & Herrington, A. (2003). Mathematics

teachers’ beliefs and curriculum reform. Mathematics

Education Research Journal, 15(1), 59–69.

https://doi.org/10.1007/BF03217369

Herrmann, Z. (2017). The Challenge of Change | Harvard

Graduate School of Education. https://www.gse.

harvard.edu/uk/blog/challenge-change

Herschbach, D. R. (2011). ERIC - EJ952045 - The STEM

Initiative: Constraints and Challenges, Journal of

sTEm Teacher Education, 2011. https://eric.ed.gov/

?id=EJ952045

Horsley, D., & Susan, L.-H. (1998). ERIC - EJ586914 -

CBAM Brings Order to the Tornado of Change.,

Journal of Staff Development, 1998. https://eric.ed.gov/

?id=EJ586914

Krajcik, J., Codere, S., Dahsah, C., Bayer, R., & Mun, K.

(2014). Planning Instruction to Meet the Intent of the

Next Generation Science Standards. Journal of Science

Teacher Education, 25(2), 157–175. https://doi.org/

10.1007/s10972-014-9383-2

Lederman, N. G., & Lederman, J. S. (2014). The Next

Generation Science Standards: Implications for

Preservice and Inservice Science Teacher Education. In

Journal of Science Teacher Education (Vol. 25, Issue

2, pp. 141–143). Kluwer Academic Publishers.

https://doi.org/10.1007/s10972-014-9382-3

Loewenberg Ball, D., Thames, M. H., & Phelps, G. (2008).

Content knowledge for teaching: What makes it

special? In Journal of Teacher Education

(Vol. 59,

Issue 5, pp. 389–407). https://doi.org/10.1177/

0022487108324554

Macmath, S., Wallace, J., & Chi, X. (2009). Problem-Based

Learning in Mathematics - A tool for Developing

Students’ Conceptual Knowledge.

Newmann Editor, F. M. (n.d.). Student Engagement and

Achievement in American Secondary Schools.

P. Lisette, Hamilton, L. S., Stecher, B. M., & Yuan, K.

(2012). Standards-Based Accountability in the United

States:: Lessons Learned and Future Directions*.

Education Inquiry, 3(2), 149–170. https://doi.org/

10.3402/edui.v3i2.22025

Paulos, J. A. (n.d.). Beyond Numeracy: Ruminations of a

Numbers Man - John Allen Paulos - Google Books.

Retrieved February 26, 2020, from

https://books.google.com/books?hl=en&lr=&id=D8K

GDwAAQBAJ&oi=fnd&pg=PR11&dq=Paulos,+J.+A

.+(1991).+Beyond+numeracy.+Vintage+Books,+NY.

+&ots=q6odDIaILC&sig=EN3r2NM3JoWQfdVhTda

hKQ6-Log#v=onepage&q&f=false

Powell-Griner, E., Anderson, J. E., & Murphy, W. (1997).

State-and sex-specific prevalence of selected

characteristics--behavioral risk factor surveillance

system, 1994 and 1995. MMWR. CDC Surveillance

Summaries : Morbidity and Mortality Weekly Report.

CDC Surveillance Summaries / Centers for Disease

Control, 46(3), 1–31.

Tarmizi, R. A., Tarmizi, M. A. A., Lojinin, N. I., &

Mokhtar, M. Z. (2010). Problem-based learning:

engaging students in acquisition of mathematical

competency. Procedia - Social and Behavioral

Sciences, 2(2), 4683–4688. https://doi.org/10.1016/

j.sbspro.2010.03.750

TRAN, C. (n.d.). Research-Based Solutions to Address

Math Anxiety. Retrieved February 26, 2020, from

https://blog.mindresearch.org/blog/math-anxiety

Trends in International Mathematics and Science Study

(TIMSS) - Overview. (n.d.). Retrieved November 19,

2019, from https://nces.ed.gov/TIMSS/

Tsupros, N., Kohler, R., & Hallinan, J. (n.d.). STEM

Education in Southwestern Pennsylvania Report of a

project to identify the missing components.

Turner, J. C., Warzon, K. B., & Christensen, A. (2011).

Motivating Mathematics Learning. American

Educational Research Journal, 48(3), 718–762.

https://doi.org/10.3102/0002831210385103

Wilson, P. S., Cooney, T. J., & Stinson, D. W. (2005). What

constitutes good mathematics teaching and how it

develops: Nine high school teachers’ perspectives.

Journal of Mathematics Teacher Education, 8(2), 83–

111. https://doi.org/10.1007/s10857-005-4796-7

WOLPERT, S. (2018). Why so many U.S. students aren’t

learning math | UCLA. http://newsroom.ucla.edu/

stories/why-so-many-u-s-students-arent-learning-math

A Study of Common Concerns Inhibiting Teacher Enactment of Computational Thinking into Project-based Mathematics and Career

Technical Education

349